8.EE.1 Teacher Guide | ilteachandtalk

EIGHT GRADE > 8.EE.1 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION

8.EE.1 APPLY THE PROPERTIES OF INTEGER EXPONENTS

COHERENCE AND CONNECTIONS

CLASSROOM RESOURCE HOT QUESTIONS ADDITIONAL RESOURCES

8.EE.1

Work with properties of integer exponents.

8.EE.1 Know and apply the properties of integer exponents to generate equivalent numerical expressions.

Apply the Properties of Integer Exponents

Students have experienced counting number exponents in grades 5 and 6. In 8th grade, they are to derive the rules from explorations. The focus of the rules is NOT procedure but equivalency. In other words, students should recognize that yields the same answer as

Instructional Strategies:

Although students begin using whole-number exponents in Grades 5 and 6, it is in Grade 8 when students are first expected to know and use the properties of exponents and to extend the meaning beyond counting-number exponents. It is no accident that these expectations are simultaneous, because it is the properties of counting-number exponents that provide the rationale for the properties of integer exponents. In other words, students should not be told these properties but rather should derive them through experience and reason.

For counting-number exponents (and for nonzero bases), the following properties follow directly from the meaning of exponents.

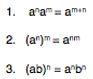
Students should have experience simplifying numerical expressions with exponents so that these properties become natural and obvious. For example:

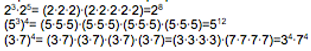
If students reason about these examples with a sense of generality about the numbers, they begin to articulate the properties. For example, “I see that 3 twos is being multiplied by 5 twos, and the results is 8 twos being multiplied together, where the 8 is the sum of 3 and 5, the number of twos in each of the original factors.” That would work for a base other than two (as long as the bases are the same).

By writing out the meaning, =3∙3∙3∙3∙3, students can see that there are only 4 multiplications.

So a better description is means 5 3s multiplied together.

Students also need to realize that these simple descriptions work only for counting number exponents. When extending the meaning of exponents to include 0 and negative exponents, these descriptions are limiting: Is it sensible to say means 0 3s multiplied together or that means 2 3’s multiplied together? Absolutely not!

Examples for deriving properties using computations:

Property 1 can be used to reason what should be.

Consider the following expression and simplification: ∙35=35. This computation shows that the when is multiplied by 35, the result (following Property 1) should be 35, which implies that must be 1. Because this reasoning holds for any base other than 0, we can reason that = 1 for any nonzero number a. To make a judgment about the meaning of , the approach is similar:

This computation shows that should be the reciprocal of , because their product is 1. And again, this reasoning holds for any nonzero base. Thus, we can reason that . Putting all of these results together, we now have the properties of integer exponents, shown the chart below.

An additional strategy for developing meaning for integer exponents is to make use of patterns, as shown in the following chart:

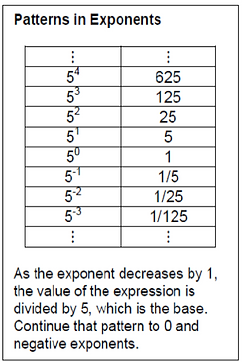
The patterns to the left, not only show that they exponents are in sequential order; but they also allow student to see the connection between the exponent, its base and its equivalency.

In addition, as the students complete the chart through division, they will realize that negative exponents create fractions as the result of division by the base.

Patterns are helpful and should be used in deriving exponent rules.

Using the place value chart is another way to show these patterns. Look at the chart below, each place in our chart is a power of ten. As you move from left to right the exponent decreases by one, as the place value is divided by ten. Use the chart then for expanded notation. This is written below the chart.

Expanded notations

Examples and Explanations

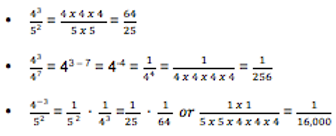
Compound exponents

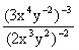
Work on either the numerator or denominator first.

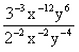
Always remove the parentheses before doing anything else. (Power Rule)

Move negative exponents to the numerator or denominator (Negative exponent rule)

Apply the product rule

Apply the quotient rule, In this case the x’s end up in the denominator because there are 10 more of them there

Finally simplify the coefficients

Kansas Association of Teachers of Mathematics (KATM) Flipbooks.

Questions or to send feedback: melisa@ksu.edu.

Retrieved from Math Flipbooks.

For additional information go to Achieve the Core.

Students learn

about rational

exponents in H.S

Coherence and Connections: Need to Know

Grade Below

Grade Level

Grade Above

6.EE.1

8.EE.1

8.EE.3

8.EE.4

A.SSE.3

A-SSE.3 states that children will be able to move fluently between exponential expressions to solve quadratic equations and determine patterns in algebra, i.e.

Illinois Assessment of Readiness Mathematics Evidence Tables https://www.isbe.net/Documents/IAR-Grade-8-Math-Evidence-State.pdf