Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
6.RP.3
7.RP.2
7.RP.3
7.SP.6
7.SP.7
7.SP.8
Illinois Assessment of Readiness Mathematics Evidence Tables https://www.isbe.net/Documents/IAR-Grade-7-Math-Evidence-State.pdf
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
7.C.4
Base explanations/reasoning on a coordinate plane diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 7.RP.A
i) Tasks use only coordinates in Quadrant 1 and use only a positive constant of proportionality.
2,3,5,6
7.C.7.1
Present solutions to multi-step problems in the form of valid chains of reasoning, using symbols such as equals signs appropriately (for example, rubrics award less than full credit for the presence of nonsense statements such as 1 + 4 = 5 + 7 = 12, even if the final answer is correct), or identify or describe errors in solutions to multi-step problems and present corrected solutions. Content Scope: Knowledge and skills articulated in 7.RP.3
i) Tasks use only coordinates in Quadrant 1 and use only a positive constant of proportionality.
1,3,6,7,8
Calculator
yes
yes
7.RP.3-1
Use proportional relationships to solve multistep ratio problems
i) Tasks will include proportional relationships that only involve positive numbers.
1,2,6
yes
7.RP.3-2
Use proportional relationships to solve multistep percent problems. Examples: simple interest, markups and markdowns, gratuities and commissions, fees, percent increase and decrease, percent error.
1,2,5,6
yes
Examples of Key Advances from Grade 6 to Grade 7
* Students grow in their ability to analyze proportional relationships. They decide whether two quantities are in a
proportional relationship (7.RP.2a); they work with percents, including simple interest, percent increase and decrease, tax, markups and markdowns, gratuities and commission, and percent error (7.RP.3); they analyze proportional
relationships and solve problems involving unit rates associated with ratios of fractions (e.g., if a person walks 1/2
mile in each 1/4 hour, the unit rate is the complex fraction ½ / ¼ miles per hour or 2 miles per hour) (7.RP.1); and they analyze proportional relationships in geometric figures (7.G.1).
Examples of Major Within-Grade Dependencies
* Meeting standard 7.EE.3 in its entirety will involve using rational number arithmetic (7.NS.1–3) and percents (7.RP.3).
Work leading to meeting this standard could be organized as a recurring activity that tracks the students’ ongoing
acquisition of new skills in rational number arithmetic and percents.
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Other lasting achievements from K-8 would include working with proportional relationships and unit rates (6.RP.3; 7.RP.1, 7.RP.2); working with percentages (6.RP.3e; 7.RP.3); and working with area, surface area, and volume (7.G.4, 6).
Also check out Student Achievement Partners Coherence Map.
Classroom Resources
Inside Mathematics video -
The clip shows a great of example of how to facilitate problem solving and daily discourse. A 19 minute clip showing students using various strategies to solve a percent problem.

Check out the student answer at minute 6:55, students were asked to think about this problem and solve mentally.
HOT Questions
This standard calls for a variety of tasks including simple interest, tax, fees, and percent errors. To reach the full depth of the standard students need to be exposed to all of these and more.
1. A shirt is on sale for 40% off. The sale price is $12.00.
a. What was the original price? $20
b. What was the amount of the discount? $8
2. Shoe Sale! I bought a pair of shoes for $59.50. I had a 15% discount. How much did I save? $10.50
3. What is 6 percent of 250? My friend used a tape diagram to solve this problem. Is she correct?
250 is 100%
25
25
25
25
25
25
25
25
25
25
Each 25 represents 10%
25/10 = 2.5
2.5 = 1%
So to find out 6 % 2.5 x 6 = 15
yes
4. If 36 grams of carbs is 12% of your daily intake. How many carbs should you have a day? 300 grams of carbs
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/102
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/105
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/106
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/117
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/121
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/130
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/132
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/148
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/266
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/765
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/884
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/886
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/997
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/1330
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/1490
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/1550
http://tasks.illustrativemathematics.org/content-standards/7/RP/A/3/tasks/2040
Inside Mathematics - Performance Tasks
https://www.insidemathematics.org/sites/default/files/materials/cereal_4.pdf
https://www.insidemathematics.org/sites/default/files/materials/lawn%20mowing_3.pdf
https://www.insidemathematics.org/sites/default/files/materials/mixing%20paints_1.pdf
https://www.insidemathematics.org/sites/default/files/materials/photographs_2.pdf
Inside Mathematics - Problem of the Month
Level C and D https://www.insidemathematics.org/sites/default/files/materials/circular%20reasoning_43.pdf
Level D https://www.insidemathematics.org/sites/default/files/materials/first%20rate_31.pdf
Level C https://www.insidemathematics.org/sites/default/files/materials/measuring%20up_37.pdf
IL Classrooms in Action - Formative Assessment
http://www.mathteachersinaction.org/formative-assessment-tasks.html
CCSStoolbox Tasks
SEVENTH GRADE > 7.RP.2 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION
7.RP.3
Analyze proportional relationships and use them to solve real-world and mathematical problems.
7.RP.3 Use proportional relationships to solve multistep ratio and percent problems. Examples: simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and decrease, percent error.
Reasoning Through Multistep Ratios and Percent Problems
This standard seems straight forward and familiar to what was done in the past. However, this standard allows teachers and students to take percent proportions a bit deeper and to an application level. Students should have plenty of practice composing and decomposing percent problems to reason through and solve for multi-step percent problems.
Let’s look at a few examples
A shirt is on sale for 40% off. The sale price is $12.00. What was the original price?
Before reading ahead, go ahead and solve this problem.
Incorrect Strategies
Students may solve this incorrectly by setting up an incorrect percent proportion.
12 = 40 This would give an incorrect answer of $30.00
40 100
Student could use a tape diagram incorrectly.

This would give an incorrect answer of $18.00 + $12.00 = $30.00 as the original price. The student did not know that $12.00 represented 60% of the original price
This is why the standard calls for proportional reasoning.
The correct answer is $20.00. Take a look at some different strategies used to solve.
12 = 60 This would give a correct answer of $20.00
60 100

That makes the 40% section worth $8.00. So, 12 + 8 = $20.00 was the original price.

Students should be able to explain the reasoning of these kinds of representations. They should be able to choose a strategy that works well for them.
Multistep problems: Students extend their work to solving multistep ratio and percent problems. 7.RP.3 Problems involving percent increase or percent decrease require careful attention to the referent whole. For example, consider the difference in these two percent decrease and percent increase problems:
Skateboard problem 1. After a 20% discount, the price of a SuperSick skateboard is $140. What was the price before the discount?
Skateboard problem 2. A SuperSick skateboard costs $140 now, but its price will go up by 20%. What will the new price be after the increase?
The solutions to these two problems are different because the 20% refers to different wholes or 100% amounts. In the first problem, the 20% is 20% of the larger pre-discount amount, whereas in the second problem, the 20% is 20% of the smaller pre-increase amount. Notice that the distributive property is implicitly involved in working with percent decrease and increase. For example, in the first problem, if x is the original price of the skateboard (in dollars), then after the 20% discount, the new price is x -20% * x. The distributive property shows that the new price is 80% * x: x - 20% * x = 100% * x - 20% * x = (100% - 20%) x = 80% * x

Once students work with these strategies, it is amazing to see how they reason through a problem using number sense. Previously, students were not given an opportunity to reason through a proportion problem. They were given the short cut or “answer getting” algorithm and they did not have options or tools to use. These strategies truly allow students to show their work vs. following the predetermined steps.

Check out the various ways students can solve and use proportional reasoning
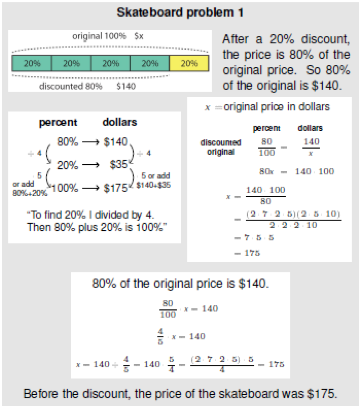


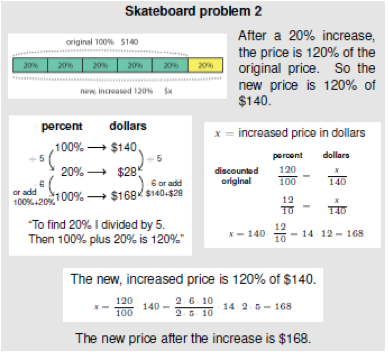

Students are solidifying their conceptual, procedural and application knowledge of ratios and proportions at this grade level. Students will be at varying levels of understanding and will need multiple ways to make proportions make sense to them (MP1).
Common Core Standards Writing Team. (2011, December 26).
Progressions for the Common Core State Standards in Mathematics(draft). 6-7, Ratios and Proportional Relationships. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona
Students should be able to explain or show their work using a representation (numbers, words, pictures, physical objects, or equations) and verify that their answer is reasonable. Models help students to identify the parts of the problem and how the values are related. For percent increase and decrease, students identify the starting value, determine the difference, and compare the difference in the two values to the starting value.
Examples:
Gas prices are projected to increase 124% by April 2015. A gallon of gas currently costs $4.17.
What is the projected cost of a gallon of gas for April 2015?
A student might say: “The original cost of a gallon of gas is $4.17. An increase of 100% means that the cost will double. I will also need to add another 24% to figure out the final projected cost of a gallon of gas. Since 25% of $4.17 is about $1.04, the projected cost of a gallon of gas should be around $9.40.”
$4.17 + $4.17 + (0.24 ⋅ 4.17) = 2.24 × 4.17
100%
100%
24%
$4.17
$4.17
?
A sweater is marked down 33%. Its original price was $37.50.
What is the price of the sweater before sales tax?
$37.50
Original Price of Sweater
33% of $37.50
67% of $37.50
Sale Price of Sweater
The discount is 33% times 37.50. The sale price of the sweater is the original price minus the discount or 67% of the original price of the sweater, or Sale Price = 0.67 x Original Price.
Additional Example Problems
At a certain store, 48 television sets were sold in April. The manager at the store wants to encourage the sales team to sell more TVs and is going to give all the sales team members a bonus if the number of TVs sold increases by 30% in May. How many TVs must the sales team sell in May to receive the bonus? Justify your solution.
A salesperson set a goal to earn $2,000 in May. He receives a base salary of $500 as well as a 10% commission for all sales. How much merchandise will he have to sell to meet his goal?
After eating at a restaurant, your bill before tax is $52.50. The sales tax rate is 8%. You decide to leave a 20% tip for the waiter based on the pre-tax amount. How much is the tip you leave for the waiter? How much will the total bill be, including tax and tip? Express your solution as a multiple of the bill.
𝑇ℎ𝑒 𝐴𝑚𝑜𝑢𝑛𝑡 𝑃𝑎𝑖𝑑=0.20×$52.50+0.08×$52.50=0.28×$52.50

Give students one of these problems and encourage them to solve it multiple ways. Another option would be to give the incorrect solution using a tape diagram strategy and have the students explain what is wrong and how to correct it.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.
