Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
5.NF.1
6.NS.5
6.NS.6a
6.NS.7c
7.NS.1
7.NS.2
7.NS.3
Fluency Expectations or Examples of Culminating Standards
7.NS.1–2 Adding, subtracting, multiplying, and dividing rational numbers is the culmination of numerical work with the four basic operations. The number system will continue to develop in grade 8, expanding to become the real numbers by the introduction of irrational numbers, and will develop further in high school, expanding to become the complex numbers with the introduction of imaginary numbers. Because there are no specific standards for rational number arithmetic in later grades and because so much other work in grade 7 depends on rational number arithmetic (see below), fluency with rational number arithmetic should be the goal in grade 7.
Examples of Major Within-Grade Dependencies
Meeting standard 7.EE.3 in its entirety will involve using rational number arithmetic (7.NS.1–3) and percents (7.RP.3). Work leading to meeting this standard could be organized as a recurring activity that tracks the students’ ongoing acquisition of new skills in rational number arithmetic and percents.
Because rational number arithmetic (7.NS.1–3) underlies the problem solving detailed in 7.EE.3 as well as the solution of linear expressions and equations (7.EE.1–2, 4), this work should likely begin at or near the start of the year.
The work leading to meeting standards 7.EE.1–4 could be divided into two phases, one centered on addition and subtraction (e.g., solving x + q = r) in relation to rational number addition and subtraction (7.NS.1) and another centered on multiplication and division (e.g., solving px + q = r and p(x + q) = r) in relation to rational number multiplication and division (7.NS.2).
Examples of Opportunities for In-Depth Focus
7.NS.3 When students work toward meeting this standard (which is closely connected to 7.NS.1 and 7.NS.2), they consolidate their skill and understanding of addition, subtraction, multiplication and division of rational numbers.
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November)
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Illinois Assessment of Readiness Mathematics Evidence Tables.
Retrieved from: https://www.isbe.net/Documents/IAR-Grade-7-Math-Evidence-State.pdf
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
7.NS.1a
Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
a. Describe situations in which opposite quantities combine to make 0. For example, a hydrogen atom has 0 charge because its two constituents are oppositely charged.
5
7.NS.1b-1
Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
b. Understand p + q as the number located a distance |q| from p, in the positive or negative direction depending on whether q is positive or negative.
i) Tasks do not have a context. ii) Tasks are not limited to integers.
iii) Tasks involve a number line.
5,7
Calculator
no
no
7.NS.1b-2
Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
b. Interpret sums of rational numbers by describing real-world contexts.
i) Tasks require students to produce or recognize real-world contexts that correspond to given sums of rational numbers.
ii) Tasks are not limited to integers.
2,3,5
no
7.NS.1c-1
Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
c. Understand subtraction of rational numbers as adding the additive inverse, p – q = p + (–q). Apply this principle in real-world contexts.
2,5,7
no
i) Tasks may or may not have a context.
ii) Tasks are not limited to integers.
iii) Contextual tasks might, for example, require students to create or identify a situation described by a specific equation of the general form p – q = p + (–q) such as 3 – 5 = 3 + (–5). iv) Non-contextual tasks are not computation tasks but rather require students to demonstrate conceptual understanding, for example, by identifying a difference that is equivalent to a given difference. For example, given the difference −1/3 − (1/5 + 5/8), the student might be asked to recognize the equivalent expression –1/3 + –(1/5 + 5/8).
7.NS.1d
Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
d. Apply properties of operations as strategies to add and subtract rational numbers
i) Tasks do not have a context. ii) Tasks are not limited to integers.
iii) Tasks may involve sums and differences of 2 or 3 rational numbers.
iv) Tasks require students to demonstrate conceptual understanding, for example, by producing or recognizing an expression equivalent to a given sum or difference. For example, given the sum −8.1 + 7.4, the student might be asked to recognize or produce the equivalent expression –(8.1 – 7.4).
5,7
no
7.C.1.1
Base explanations/reasoning on the properties of operations. Content Scope: Knowledge and skills articulated in 7.NS.1 and 7.NS.2
) Tasks should not require students to identify or name properties.
1,2,3,5,
6,7
yes
7.C.2
Base explanations/reasoning on the relationship between addition and subtraction or the relationship between multiplication and division. Content Scope: Knowledge and skills articulated in 7.NS.1 and 7.NS.2
1,2,3,5,
6,7
yes
7.C.3
Base explanations/reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 7.NS.A
1,2,3,5,
6,7
yes
Also check out Student Achievement Partners Coherence Map.
HOT Questions
1. Rewrite the expression as an addition problem.
(-3) – 5
2. If you subtract a negative from a positive, will your answer always be positive? Explain how you know.
3. Use a drawing or model to add the two negative integers.
4. Write the addition problem of
5. (-5) +( -15) or ( - 5) – ( -15)
Which is larger?
Which expression covers a greater distance on a number line?
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/46
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/310
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/314
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/317
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/591
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/998
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/1475
http://tasks.illustrativemathematics.org/content-standards/7/NS/A/1/tasks/1987
Inside Mathematics - Problem of the Month
Level D https://www.insidemathematics.org/sites/default/files/materials/fractured%20numbers_40.pdf
Level E https://www.insidemathematics.org/sites/default/files/materials/part%20and%20whole_24.pdf
Level D https://www.insidemathematics.org/sites/default/files/materials/diminishing%20return_49.pdf
Engage NY Module – scroll down to student materials
https://www.engageny.org/resource/grade-7-mathematics-module-2
SEVENTH GRADE > 7.NS.1 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION
7.NS.1
Apply and extend previous understandings of operations with fractions to add, subtract, multiply and divide rational numbers.
7.NS.1 Apply and extend previous understandings of addition and subtraction to add and subtract rational numbers; represent addition and subtraction on a horizontal or vertical number line diagram.
a. Describe situations in which opposite quantities combine to make 0. For example, a hydrogen atom has 0 charge because its two constituents are oppositely charged.
b. Understand p + q as the number located a distance |q| from p, in the positive or negative direction depending on whether q is positive or negative. Show that a number and its opposite have a sum of 0 (are additive inverses). Interpret sums of rational numbers by describing real-world contexts.
c. Understand subtraction of rational numbers as adding the additive inverse, p - q = p + (-q). Show that the distance between two rational numbers on the number line is the absolute value of their difference, and apply this principle in real-world contexts.
d. Apply properties of operations as strategies to add and subtract rational numbers.
Add and Subtract Rational Numbers
This standard calls for students to apply and extend understandings of addition and subtraction to rational numbers. Notice in the subsets of the standard understanding and application is explicitly listed and describes how to take this concept beyond the procedural.
Students will: Add and subtract rational numbers
-
Represent addition and subtraction on a horizontal and vertical number line
-
Understand p + q as the number located a distance |q| from , points on a number line show distance and direction
-
Interpret sums of rational numbers by describing real-world contexts.
-
Understand subtraction of rational numbers as adding the additive inverse
-
Apply properties of operations as strategies to add and subtract rational numbers
Progression Document NS
Grade 7
Addition and subtraction of rational numbers. In Grade 6 students learned to locate rational numbers on the number line; in Grade 7 they extend their understanding of operations with fractions to operations with rational numbers.

Whereas previously addition was represented by concatenating (link together) the line segments together, now the line segments have directions, and therefore a beginning and an end.
When concatenating these directed line segments, we start the second line segment at the end of the first one. If the second line segment is going in the opposite direction to the first, it can backtrack over the first, effectively cancelling part or all of it out.7.NS.1b Later in high school, if students encounter vectors, they will be able to see this as one-dimensional vector addition.
A fundamental fact about addition of rational numbers is that p+ (-p) = 0 for any rational number p; in fact, this is a new property of operations that comes into play when negative numbers are introduced. This property can be introduced using situations in which the equation makes sense in a context. 7.NS.1a

* Represent addition and subtraction on a horizontal and vertical number line
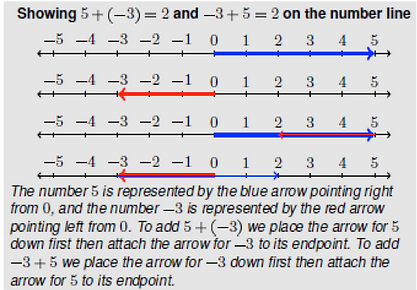
For example, the operation of adding an integer could be modeled by an elevator moving up or down a certain number of floors. It can also be shown using the directed line segment model of addition on the number, as shown in the margin.7.NS.1b

The addition and subtraction of integers is sometimes a difficult concept. Students need time to explore this concept and not just follow a set of rules. Encourage discovery learning in order to find integer rules.
It is common to use colored chips to represent integers, with one color representing positive integers and another representing negative integers, subject to the rule that chips of different colors cancel each other out; thus, a number is not changed if you take away or add such a pair. This is rather a different representation than the number line. On the number line, the equation p+ (-p) = 0 follows from the definition of addition using directed line segments.
With integer chips, the equation p + (-p) = 0 is true by definition since it is encoded in the rules for manipulating the chips. Also implicit in the use of chips is that the commutative and associative properties extend to addition of integers, since combining chips can be done in any order.
However, the integer chips are not suited to representing rational numbers that are not integers. Whether such chips are used or not, the Standards require that students eventually understand location and addition of rational numbers on the number line. With the number line model, showing that the properties of operations extend to rational numbers requires some reasoning. Although it is not appropriate in Grade 6 to insist that all the properties be proved to hold in the number line or chips model, experimenting with them in these models is a good venue for reasoning (MP.2).7.NS.1d



Understanding direction and distance on the number line


Subtraction of rational numbers is defined the same way as for positive rational numbers: p – q is defined to be the missing addend in q + ? = p. For example, in earlier grades, students understand 5 - 3 as the missing addend in 3 + ? = 5. On the number line, it is represented as the distance from 3 to 5. Or, with our new found emphasis on direction on the number line, we might say that it is how you get from 3 from 5; by going two units to the right (that is, by adding 2).
In Grade 6 students apply the same understanding to (-5) – (-3). It is the missing addend in
(-3) + ? = -5, or how you get from -3 to -5. Since -5 is two units to the left of -3 on the number line, the missing addend is -2.

Review this concept in grade 7, just in case students did not get it in grade 6.
What addition problem can you make?
5 – (-3)
(-3) – 7
(-7) – (-2)
How do you know? Or Show on a number line.

The idea behind direction and distance on a number line is that:
+ means moving right
- can mean moving left or the opposite of the previous sign
For example,
6 + (-7)
Would be interpreted as “start at positive 6”
+ means get ready to move right
-7 means to go the opposite of right which is left 7
This would land you on -1
6 – (-7)
Would be interpreted as “start at positive 6”
- means get ready to head left
-7 means go the opposite 7 or head to the right
Th on 13
* Understand subtraction of rational numbers as adding the additive inverse
With the introduction of direction on the number line, there is a distinction between the distance from a and b and how you get from a to b. The distance from -3 to -5 is 2 units, but the instructions how to get from -3 to -5 are “go two units to the left.” The distance is a positive number, 2, whereas “how to get there” is a negative number -2. In Grade 6 we introduce the idea of absolute value to talk about the size of a number, regardless of its sign. It is always a positive number or zero. If p is positive, then its absolute value is just p; if p is negative then = -p . With this interpretation we can say that the absolute value of p - q is just the distance from p to q, regardless of direction.7.NS.1c

Understanding p - q as a missing addend also helps us see that p + (-q) = p – q ).7.NS.1c Indeed, p – q is the missing number in q + ? = p and p + (-q) satisfies the description of being that missing, number:
q + (p + (-q)) = p + (q + (-q)) = p + 0 = p
The figure in the margin illustrates this in the case where p and q are positive and p > q.

* Interpret sums of rational numbers by describing real-world contexts.
Common Core Standards Writing Team. (2013, July 4).
Progressions for the Common Core State Standards in Mathematics (draft). Grades 6–8, The Number System; High School, Number. Tucson, AZ: Institute for Mathematics and Education, University of Arizona.
The temperature yesterday was 55° degrees. A cold front has moved in and the temperature has dropped 17° degrees. Write 2 equations that represent the current temperature. What is the current temperature?
Possible Answer
55 + (-17) =
(-17) + 55 =
55 – 17 =
The new temperature is 38° degrees
Write a word problem and equation that represents the number line below.
Word Problem Example
The wind chill factor was reading -2° degrees, then the winds stopped and the temperature was two degrees above zero. Later that evening the temperature dropped 6° degrees. What is the current temperature?
(-2) + 4 + (-6)
(-2) + 4 – 6
Explanations and Examples:
Students add and subtract rational numbers using a number line. For example, to add -5 + 7, students would find -5 on the number line and move 7 in a positive direction (to the right). The stopping point of 2 is the sum of this expression.
Students also add negative fractions and decimals and interpret solutions in given contexts.
Visual representations may be helpful as students begin this work; they become less necessary as students become more fluent with the operations.
Examples:
Use a number line to illustrate:
𝑝−𝑞
𝑝+(−𝑞)
Is this equation true: 𝑝−𝑞=𝑝+(−𝑞)
-3 and 3 are shown to be opposites on the number line because they are equal distance from zero and therefore have the same absolute value and the sum of the number and it’s opposite is zero.

You have $4 and you need to pay a friend $3. What will you have after paying your friend?
4 + (-3) = 1 or (-3) + 4 = 1

Name a number that makes each statement true. Justify your solution by showing a model or providing an explanation.
-
−4.8 + ? = 𝑎 𝑝𝑜𝑠𝑖𝑡𝑖𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟
-
? – 3 2= 𝑎 𝑛𝑒𝑔𝑎𝑖𝑡𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟
-
−2.15 − ? = 𝑎 𝑛𝑒𝑔𝑎𝑡𝑖𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟
Instructional Strategies:
This cluster (7.NS.1-3) builds upon the understandings of rational numbers in Grade 6:
-
quantities can be shown using + or – as having opposite directions or values,
-
points on a number line show distance and direction,
-
opposite signs of numbers indicate locations on opposite sides of 0 on the number line,
-
the opposite of an opposite is the number itself,
-
the absolute value of a rational number is its distance from 0 on the number line,
-
the absolute value is the magnitude for a positive or negative quantity, and
-
locating and comparing locations on a coordinate grid by using negative and positive numbers.
Learning now moves to exploring and ultimately formalizing rules for operations (addition, subtraction, multiplication and division) with integers. Using both contextual and numerical problems, students should explore what happens when negatives and positives are combined.
Number lines present a visual image for students to explore and record addition and subtraction results.
Fractional rational numbers and whole numbers should be used in computations and explorations.
Students should be able to give contextual examples of integer operations, write and solve equations for real- world problems and explain how the properties of operations apply. Real-world situations could include: profit/loss, money, weight, sea level, debit/credit, football yardage, etc.
Using what students already know about positive and negative whole numbers and multiplication with its relationship to division, students should generalize rules for multiplying and dividing rational numbers. Multiply or divide the same as for positive numbers, then designate the sign according to the number of negative factors. Students should analyze and solve problems leading to the generalization of the rules for operations with integers. For example, beginning with known facts, students predict the answers for related facts, keeping in mind that the properties of operations apply (See Tables 1, 2 and 3 below).

Using the language of “the opposite of” helps some students understand the multiplication of negatively signed numbers ( −4×−4=16, the opposite of 4 groups of -4). Discussion about the tables should address the patterns in the products, the role of the signs in the products and commutativity of multiplication. Then students should be asked to answer these questions and prove their responses:
-
Is it always true that multiplying a negative factor by a positive factor results in a negative product?
-
Does a positive factor times a positive factor always result in a positive product?
-
What is the sign of the product of two negative factors?
-
When three factors are multiplied, how is the sign of the product determined?
- How is the numerical value of the product of any two numbers found?


Encourage Student Discovery Learning
Let them explore, persevere, explain, model and discover rules of integers
Students can use number lines with arrows and hops, groups of colored chips or logic to explain their reasoning. When using number lines, establishing which factor will represent the length, number and direction of the hops will facilitate understanding. Through discussion, generalization of the rules for multiplying integers would result.
Division of integers is best understood by relating division to multiplication and applying the rules. In time, students will transfer the rules to division situations. (Note: In 2b, this algebraic language (−(𝑝𝑞)=(−𝑝)𝑞=𝑝(−𝑞)) is written for the teacher’s information, not as an expectation for students.)
Ultimately, students should solve other mathematical and real-world problems requiring the application of these rules with fractions and decimals.
In Grade 7 the awareness of rational and irrational numbers is initiated by observing the result of changing fractions to decimals. Students should be provided with families of fractions, such as, sevenths, ninths, thirds, etc. to convert to decimals using long division. The equivalents can be grouped and named (terminating or repeating). Students should begin to see why these patterns occur. Knowing the formal vocabulary rational and irrational is not expected.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
For additional information go to Achieve the Core.
