Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
6.EE.3
6.EE.4
7.EE.1
7.EE.2
Examples of Key Advances from Grades K–8
Themes beginning in middle school algebra continue and deepen during high school. As early as grades 6 and 7, students began to use the properties of operations to generate equivalent expressions (6.EE.3, 7.EE.1). By grade 7, they began to recognize that rewriting expressions in different forms could be useful in problem solving (7.EE.2). In Algebra I, these aspects of algebra carry forward as students continue to use properties of operations to rewrite expressions, gaining fluency and engaging in what has been called “mindful manipulation.” 26
26 See, for example, “Mindful Manipulation,” in Focus in High School Mathematics: Reasoning and Sense Making (National Council of Teachers of Mathematics, 2009).
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November). Retrieved from:
https://files.eric.ed.gov/fulltext/ED582070.pdf
Illinois Assessment of Readiness Mathematics Evidence Tables. Retrieved from:
https://www.isbe.net/Documents/IAR-Grade-7-Math-Evidence-State.pdf
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
7.EE.2
Understand that rewriting an expression in different forms in a problem context can shed light on the problem and how the quantities in it are related. For example, a + 0.05a = 1.05a means that "increase by 5%" is the same as "multiply by 1.05."
7
Calculator
no
Also check out Student Achievement Partners Coherence Map.
HOT Questions
1. a. Is x2 the same as x2 +1 -1?
Yes
b. Write another expression for x2.
2(x2) / 2
2. Malia is at an amusement park. She bought 14 tickets, and each ride requires 2 tickets.
a. Write an expression that gives the number of tickets Malia has left in terms of the number of rides she has already
gone on. Find at least one other expression that is equivalent to it.
Possible solutions
14−2x
2(7−x)
14−x−x
10−2x+4
−2x+14
b. 14 − 2x represent the number of tickets Malia has left after she has gone on rides. How can each of the following
numbers and expressions be interpreted in terms of tickets and rides?
14
-2
2x
In the expression 14−2x, the 14 represents the number of tickets Malia started with since the value of the expression is 14 when x=0. The −2 represents the number of tickets she spends per ride. 2x represents the number of tickets she has
to subtract from her initial amount after riding x rides.
c. 2(7 − x) also represent the number of tickets Malia has left after she has gone on rides. How can each of the following
numbers and expressions be interpreted in terms of tickets and rides?
7
(7 − x)
2
In the expression 2(7−x), the 7 represents the total number of rides Malia can go on. (7−x) represents the number of
rides she has left and the 2 represents the number of tickets required for each ride Malia has left.
http://tasks.illustrativemathematics.org/content-standards/7/EE/A/2/tasks/1450
3. The store charges 50% more than what they pay for all their merchandise.
Which expression can be used to calculate what they should charge the customers?
a. 0.5x
b. 1.5x
c. 50x
d. 150x
b
Additional Resources
Inside Mathematics - Problem of the Month
https://www.insidemathematics.org/sites/default/files/materials/the%20wheel%20shop_37.pdf
Mathematics Assessment Project
http://www.map.mathshell.org.uk/materials/tasks.php?taskid=399&subpage=novice
Georgia Department of Education
https://www.georgiastandards.org/Georgia-Standards/Frameworks/7th-Math-Unit-2.pdf
Howard County
SEVENTH GRADE > 7.EE.2 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION
7.EE.2
Use properties of operations to generate equivalent expressions
7.EE.2 Understand that rewriting an expression in different forms in a problem context can shed light on the problem and how the quantities in it are related. For example, a + 0.05a = 1.05a means that “increase by 5%” is the same as “multiply by 1.05.”
Rewriting Expressions
Students should be allowed to explore more complex expressions and rewrite equivalent expressions. It is important to provide word problems and pictures that allow for students to represent the problem in a variety of ways.
Have daily discussions about the different ways in which students can represent an expression.
Example:

How many squares will be in the 5th and 6th LOGO? How many squares will be in the 100th LOGO? How many squares will be in the nth LOGO? Please explain how your pattern works.
Utah Common Core Academy http://eucc2011.wikispaces.com/7th+Grade+Mathematics

Students could represent this pattern in a variety of ways.
Example n + (n +1) + ( n + 1)
Explanations and Examples:
Students understand the reason for rewriting an expression in terms of a contextual situation. For example, students understand that a 20% discount is the same as finding 80% of the cost (.80c). All varieties of a brand of cookies are $3.50. A person buys 2 peanut butter, 3 sugar and 1 chocolate. Instead of multiplying 2 x $3.50 to get the cost of the peanut butter cookies, 3 x $3.50 to get the cost of the sugar cookies and 1 x $3.50 for the chocolate cookies and then adding those totals together. Students recognize that multiplying $3.50 times 6 will give the same total.
Examples:
Jamie and Ted both get paid an equal hourly wage of $9 per hour. This week, Ted made an additional $27 dollars in overtime. Write an expression that represents the weekly wages of both if J = the number of hours that Jamie worked this week and T = the number of hours Ted worked this week? Can you write the expression in another way?
Students may create several different expressions depending upon how they group the quantities in the problem. Possible student responses:
1. To find the total wage, I would first multiply the number of hours Jamie worked by 9. Then I would multiply the
number of hours Ted worked by 9. I would add these two values with the $27 overtime to find the total wages for the week. The student would write the expression.
9J + 9T + 27
2. To find the total wages, I would add the number of hours that Ted and Jamie worked. I would multiply the total
number of hours worked by 9. I would then add the overtime to that value to get the total wages for the week. The
student would write the expression
9(J + T) + 27
3. To find the total wages, I would need to figure out how much Jamie made and add that to how much Ted made
for the week. To figure out Jamie’s wages, I would multiply the number of hours she worked by 9. To figure out
Ted’s wages, I would multiply the number of hours he worked by 9 and then add the $27 he earned in overtime.
My final step would be to add Jamie and Ted wages for the week to find their combined total wages. The student
would write the expression
(9J) + (9T + 27)

Allow students to showcase and explain the different ways to represent the real world problem with an expression
Given a square pool as shown in the picture, write four different expressions to find the total number of tiles in the border. Explain how each of the expressions relates to the diagram and demonstrate that the expressions are equivalent.
Which expression do you think is most useful? Explain your thinking.

Instructional Strategies: 7.EE.3; (See 7.EE.1)
Provide opportunities for students to experience expressions for amounts of increase and decrease. In Standard 2, the expression is rewritten and the variable has a different coefficient. In context, the coefficient aids in the understanding of the situation. Another example is this situation which represents a 10% decrease: b - 0.10b = 1.00b - 0.10b which equals 0.90b or 90% of the amount.
Common Misconceptions:
As students begin to build and work with expressions containing more than two operations, students tend to set aside the order of operations.
Example
Tom wants to save 30% of the money he makes delivering pizza. He made d dollars last week. How can he figure out how much money to keep?
0.70d
d - (0.30d)
For example having a student simplify an expression like 8 + 4(2x - 5) + 3x can bring to light several misconceptions.
-
Do the students immediately add the 8 and 4 before distributing the 4?
-
Do they only multiply the 4 and the 2x and not distribute the 4 to both
terms in the parenthesis?
-
Do they collect all like terms 8 + 4 – 5, and 2x + 3x?
Each of these show gaps in students’ understanding of how to simplify numerical expressions with multiple operations.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
In contrast with the simple linear expressions they see in Grade 6, the more complex expressions students seen in Grade 7 afford shifts of perspective, particularly because of their experience with negative numbers: for example, students might see 7 – 2 (3 - 8x) as 7 – (2 (3 – 8x)) or as 7 + (-2) (3 + (-8) x) (MP7).
As students gain experience with multiple ways of writing an expression, they also learn that different ways of writing expressions can serve different purposes and provide different ways of seeing a problem. For example, a + 0.05a =1.05a means that “increase by 5%” is the same as “multiply by 1.05.”7.EE.2 In the example below , the connection between the expressions and the figure emphasize that they all represent the same number, and the connection between the structure of each expression and a method of calculation emphasize the fact that expressions are built up from operations on numbers.
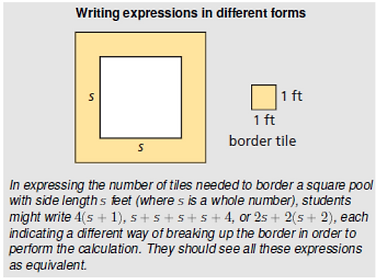

This standard goes beyond just simplifying expressions. Students need to learn “mindful manipulation” in order to set the foundation for Algebra
Common Core Standards Writing Team. (2011, April 22).
Progressions for the Common Core State Standards in Mathematics(draft). 6-8, Expressions and Equations. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.
