TEACHER GUIDE TO CLARIFICATION
Finding Distances between Points on a Coordinate Plane
Students find the distance between points whose ordered pairs have the same x- coordinate (vertical) or same y-coordinate (horizontal).
For example, the distance between (–5, 2) and (–9, 2) would be 4 units. This would be a horizontal line since the y-coordinates are the same. In this scenario, both coordinates are in the same quadrant.

Allow students to explore all of the characteristics of these points. For example, the distance is 4 units, the line would be horizontal and that both coordinates are in the same quadrant.
The distance can be found by using a number line to find the distance between – 5 and – 9. Students could also recognize that –5 is 5 units from 0 (absolute value) and that –9 is 9 units from 0 (absolute value). Since both of these are in the same quadrant, the distance can be found by finding the difference between 9 and 5. (| 9 | - | 5 |).
Coordinates could also be in two quadrants. For example, the distance between (3, –5) and (3, 7) would be 12 units. This would be a vertical line since the x-coordinates are the same. The distance can be found by using a number line to count from –5 to 7 or by recognizing that the distance (absolute value) from –5 to 0 is 5 units and the distance (absolute value) from 0 to 7 is 7 units so the total distance would be 5 + 7 or 12 units. Students graph coordinates for polygons and find missing vertices based on properties of triangles and quadrilaterals
Example:
* If the points on the coordinate plane below are the three vertices of a rectangle, what are the coordinates of the
fourth vertex? How do you know? What are the length and width of the rectangle?

Points can be in two quadrants, requiring students to
apply absolute value to find distance.
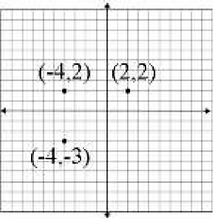
To determine the distance along the x-axis between the point (-4, 2) and (2, 2) a student must recognize that -4 is |-4| or 4 units to the left of 0 and 2 is |2| or 2 units to the right of zero, so the two points are total of 6 units apart along the x-axis. Students should represent this on the coordinate grid and numerically with an absolute value expression, |-4| + |2|.
Instructional Strategies NS. 5 through 8
The purpose of this cluster (NS 5-8) is to begin study of the existence of negative numbers, their relationship to positive numbers, and the meaning and uses of absolute value. Starting with examples of having/owing and above/below zero sets the stage for understanding that there is a mathematical way to describe opposites. Students should already be familiar with the counting numbers (positive whole numbers and zero), as well as with fractions and decimals (also positive). They are now ready to understand that all numbers have an opposite. These special numbers can be shown on vertical or horizontal number lines, which then can be used to solve simple problems. Demonstration of understanding of positives and negatives involves translating among words, numbers and models: given the words “7 degrees below zero,” showing it on a thermometer and writing -7; given -4 on a number line, writing a real-life example and mathematically -4. Number lines also give the opportunity to model absolute value as the distance from zero.
Simple comparisons can be made and order determined. Order can also be established and written mathematically: -3° C > -5° C or -5° C < -3° C. Finally, absolute values should be used to relate contextual problems to their meanings and solutions.
Using number lines to model negative numbers, prove the distance between opposites, and understand the meaning of absolute value easily transfers to the creation and usage of four- quadrant coordinate grids. Points can now be plotted in all four quadrants of a coordinate grid. Differences between numbers can be found by counting the distance between numbers on the grid. Actual computation with negatives and positives is handled in Grade 7.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
Grade 6 PARCC Performance Based Assessment Computer-Based Practice Test
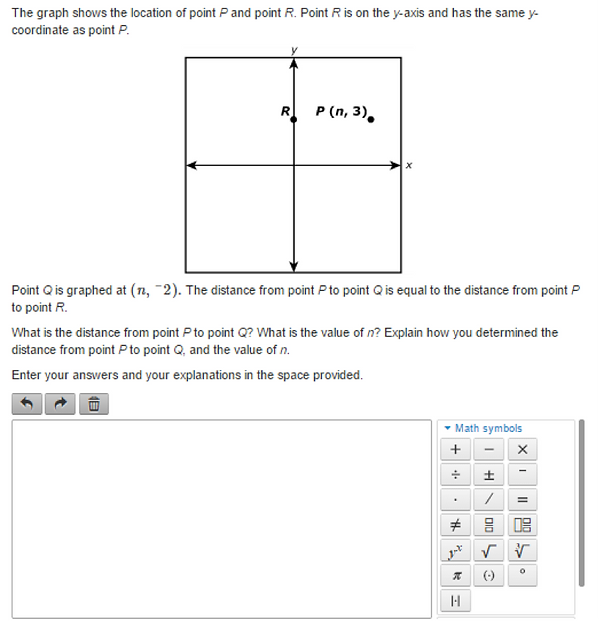

Notice the use of a variable.
Provide opportunity to practice these kinds of situations.
Rubric for Answer
Student response includes each of the following 4 elements:
-
Correct distance from point P to point Q, 5
-
Valid explanation for determining the distance from point P to point Q
-
Valid explanation for determining the value of n
-
Correct value for n, 5
Sample Student Response:
The distance from point P to point Q is 5 units because point P is 3 units above the x-axis. Point Q is 2 units below the x-axis. So 3+2=5.
Point Q is 5 units below point P, therefore the distance from point P to point R is also 5 units. Since R is on the y-axis, it has an x-coordinate of 0. So the x-coordinate of point P is 5 units to the right and is 5.
The value for n is 5.
Grade 6 PARCC End Of Year Computer-Based Practice Test


Solve real-world mathematical problems by graphing

6.NS.8
Apply and extend previous understandings of numbers to the system of rational numbers.
6.NS.8 Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinate and absolute value to find distances between points with the same first coordinate or the same second coordinate.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
5.G.2
6.NS.6b
6.NS.8
6.G.3
8.SP.1
Examples of Major Within Grade Dependencies
Students must be able to place rational numbers on a number line (6.NS.7) before they can place ordered pairs of rational numbers on a coordinate plane (6.NS.8). The former standard about ordering rational numbers is much more fundamental.
Examples of Opportunities for Connections among Standards, Clusters or Domains
Plotting rational numbers in the coordinate plane (6.NS.8) is part of analyzing proportional relationships (6.RP.3a, 7.RP.2) and will become important for studying linear equations (8.EE.8) and graphs of functions (8.F).
Examples for Opportunities for In-Depth Focus
6.NS.8 When students work with rational numbers in the coordinate plane to solve problems, they combine and consolidate elements from the other standards in this cluster.
Continue to stress the proportional relationship when plotting rational numbers on a coordinate plane.
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Although negative integers might be commonly used as initial examples of negative numbers, the Standards do not introduce the integers separately from the entire system of rational numbers, and examples of negative fractions or decimals can be introduced from the beginning.

Once rational numbers are introduced in 6th grade (6.NS.6) all rational numbers can be used with any given standard.
Illinois Assessment of Readiness Mathematics Evidence Tables: https://www.isbe.net/Documents/IAR-Grade-6-Math-Evidence-State.pdf
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
6.C.5
Base explanations/reasoning on a coordinate plane diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 6.NS.6, 6.NS.8
6.NS.8
Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinates and absolute value to find distances between points with the same first coordinate or the same second coordinate.
i) Tasks may or may not contain context.
ii) Finding distances is limited to points with integer coordinates.
1,2,5
yes
Calculator
no
3,4,5,6
Also check out Student Achievement Partners Coherence Map.
HOT Questions
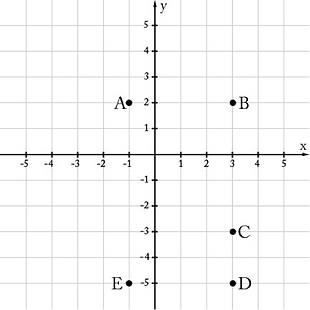

Use this graph for
Questions 1 - 3
1. Which of the points shown above are 4 units away from (−1,−3) and 2 units away from (3,−1) ?
Point C
2. Name two coordinate points that form a horizontal line. What do you notice about each set of points?
The y-value is the same.
3. Use two different strategies to determine the distance between E and D.
Example – Use the graph to count the units horizontally. 4 units
Set up an equation I-1I + 3 = 4 units
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/8/tasks/290
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/8/tasks/2221
EngageNY – 6.NS.8
https://www.engageny.org/ccls-math/6ns8
Howard County
