TEACHER GUIDE TO CLARIFICATION
Represent All Rational Numbers on Number lines and Graphs
In 6th grade students will build upon their knowledge of rational numbers and be apply to represent points on the number line and in a coordinate plane with negative coordinates.
Students will:
-
Understand a Rational Number as a Point
-
Understand Signs of Numbers in Ordered Pairs
-
Plot coordinate positions on a graph
6.NS.6 In elementary school, students worked with positive fractions, decimals and whole numbers on the number line. In 6th grade, students extend the number line to represent all rational numbers and recognize that number lines may be either horizontal or vertical (i.e. thermometer). Students recognize that a number and its opposite are equidistance from zero (reflections about the zero). The opposite sign (–) shifts the number to the opposite side of 0. For example, – 4 could be read as “the opposite of 4” which would be negative 4. The following example, – (–6.4) would be read as “the opposite of the opposite of 6.4” which would be 6.4. Zero is its own opposite.

Understanding this idea of distance and direction on a number line is a critical skill.
-
Students worked with Quadrant I in elementary school. As the x-axis and y-axis are extending to include negatives, students begin with the Cartesian Coordinate system. Students recognize the point where the x-axis and y-axis intersect as the origin.
-
Students identify the four quadrants and are able to identify the quadrant for an ordered pair based on the signs of the coordinates. For example, students recognize that in Quadrant II, the signs of all ordered pairs would be (–, +).
Ex. Question - What would be the signs of all ordered pairs in Quadrant III?
-
Students understand the relationship between two ordered pairs differing only by signs as reflections across one or both axes. For example, in the ordered pairs (-2, 4) and (-2, -4), the y-coordinates differ only by signs, which represents a reflection across the x-axis. A change is the x-coordinates from (-2, 4) to (2, 4), represents a reflection across the y-axis. When the signs of both coordinates change, [(2, -4) changes to (-2, 4)], the ordered pair has been reflected across both axes.

Students should have time exploring these different skills and coming to conclusions on their own. Discovery learning is encouraged and then practiced for mastery.
-
Students are able to plot all rational numbers on a number line (either vertical or horizontal) or identify the values of given points on a number line. For example, students are able to identify where the following numbers would be on a number line: –4.5, 2, 3.2, –3 3/5, 0.2, –2, 11/2
-
Number lines can be used to show numbers and their opposites. Both 3 and -3 are 3 units from zero on the number line. Graphing points and reflecting across zero on a number line extends to graphing and reflecting points across axes on a coordinate grid. The use of both horizontal and vertical number line models facilitates the movement from number lines to coordinate grids.

Fluency
Definition
Example 1:
-
Graph the following points in the correct quadrant of the coordinate plane. If you reflected each point across the x-axis, what are the coordinates of the reflected points? What similarities do you notice between coordinates of the original point and the reflected point?
Solution:
The coordinates of the reflected points would be (1/2, 3 1/2) (-1/2, 3) (0.25, -.75) Note that the y-coordinates are opposite.
Example 2:
What is the opposite of 2 ½? Explain your answer?
Solution:
-2 ½ because it is the same distance from 0 on the opposite side.
Students place the following numbers would be on a number line: -4.5, 2, 3.2, -3 3/5, 0.2, -2, 11/2. Based on number line placement, numbers can be placed in order.
Solution:
The numbers in order from least to greatest are:
-4.4, -3 3/5, -2, 0.2, 2, 3.2, 11/2.
Students place each of these numbers on a number line to justify this order.
Instructional Strategies NS. 5 through 8
The purpose of this cluster (NS 5-8) is to begin study of the existence of negative numbers, their relationship to positive numbers, and the meaning and uses of absolute value. Starting with examples of having/owing and above/below zero sets the stage for understanding that there is a mathematical way to describe opposites. Students should already be familiar with the counting numbers (positive whole numbers and zero), as well as with fractions and decimals (also positive). They are now ready to understand that all numbers have an opposite. These special numbers can be shown on vertical or horizontal number lines, which then can be used to solve simple problems. Demonstration of understanding of positives and negatives involves translating among words, numbers and models: given the words “7 degrees below zero,” showing it on a thermometer and writing -7; given -4 on a number line, writing a real-life example and mathematically -4. Number lines also give the opportunity to model absolute value as the distance from zero.
Simple comparisons can be made and order determined. Order can also be established and written mathematically: -3° C > -5° C or -5° C < -3° C. Finally, absolute values should be used to relate contextual problems to their meanings and solutions.
Using number lines to model negative numbers, prove the distance between opposites, and understand the meaning of absolute value easily transfers to the creation and usage of four- quadrant coordinate grids. Points can now be plotted
in all four quadrants of a coordinate grid. Differences between numbers can be found by counting the distance between numbers on the grid. Actual computation with negatives and positives is handled in Grade 7.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
Grade 6 PARCC Performance Based Assessment Computer-Based Practice Test


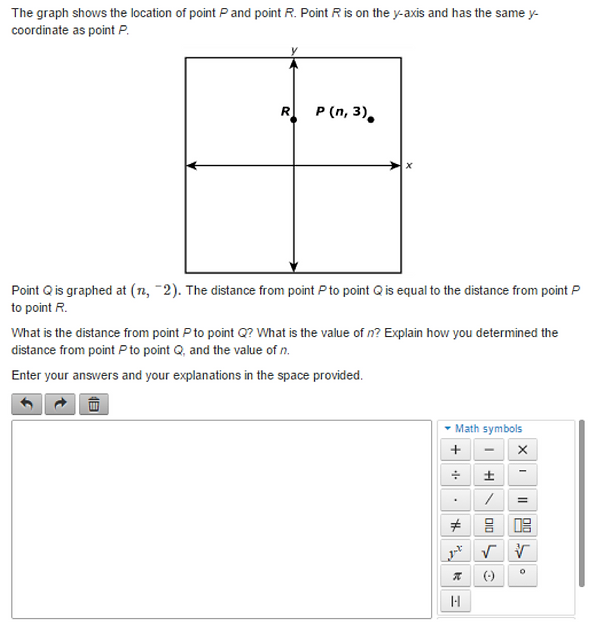
Grade 6 PARCC End Of Year Computer-Based Practice Test

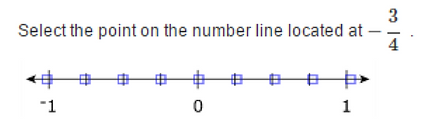
For additional information go to Achieve the Core.

6.NS.6
Apply and extend previous understandings of numbers to the system of rational numbers.
6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates.
-
Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., -(-3)=3, and that 0 is its own opposite.
-
Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only in signs, the locations of the points are related by reflections across one or both axes.
-
Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
3.NF.2
5.G.1
6.NS.5
6.NS.6
6.NS.7
6.NS.8
6.EE.8
7.NS.1a,b
Connections:
This cluster does not directly address one of the Grade 6 Critical Areas of Focus. However, it is the foundation for working with rational numbers, algebraic expressions and equations, functions, and the coordinate plane in subsequent grades.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
Examples of Key Advances
Students extend their work with the system of rational numbers to include using positive and negative numbers to describe quantities (6.NS.5), extending the number line and coordinate plane to represent rational numbers and ordered pairs (6.NS.6), and understanding ordering and absolute value of rational numbers (6.NS.7).
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Although negative integers might be commonly used as initial examples of negative numbers, the Standards do not introduce the integers separately from the entire system of rational numbers, and examples of negative fractions or decimals can be introduced from the beginning.
Directed measurement scales for temperature and elevation provide a basis for understanding positive or negative direction on the number line (6.NS.6a). Previous understanding of numbers on the number line related the position of the number to measurement: the number 5 is located at the endpoint of a line segment 5 unit long whose other endpoint is at 0. Now the line segments acquire direction: starting at 0 they can go in either the positive or negative direction. These directed numbers can be represented by putting arrows at the endpoints of the line segments.

Students come to see -as the opposite of, located an equal distance from 0 in the opposite direction. In order to avoid the common misconception later in algebra that any symbol with a negative sign in front of it should be a negative number, it is useful for students to see examples where -p is a positive number, for example if p = -3 then -p = -(-3) = 3. Students come to see the operation of putting a negative sign in front of a number as flipping the direction of the number from positive to negative or negative to positive. Students generalize this understanding of the meaning of the negative sign to the coordinate plane, and can use it in locating numbers on the number line and ordered pairs in the coordinate plane (6.NS.6bc).
Stress Direction

Common Core Standards Writing Team. (2013, July 4).
Progressions for the Common Core State Standards in Mathematics (draft). Grades 6–8, The Number System; High School, Number. Tucson, AZ: Institute for Mathematics and Education, University of Arizona.
Illinois Assessment of Readiness Mathematics Evidence Tables:
https://www.isbe.net/Documents/IAR-Grade-6-Math-Evidence-State.pdf
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
6.NS.6a
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite.
i) Tasks have “thin context”1 or no context.
6.NS.6b-1
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane
i) Tasks have” thin context” or no context.
ii) Students need not recognize or use traditional notation for quadrants (such as I, II, III, IV).
iii) Coordinates are not limited to integers.
5
no
Calculator
no
5,8
6.NS.6b-2
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. b. Recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes.
i) Tasks have” thin context” or no context.
ii) Students need not recognize or use traditional notation for quadrants (such as I, II, III, IV).
iii) Coordinates are not limited to integers.
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane
no
5,8
6.NS.6c-1
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram.
i) Tasks have” thin context” or no context.
ii) Coordinates are not limited to integers.
no
5,8
6.NS.6c-2
Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. c. Find and position pairs of integers and other rational numbers on a coordinate plane.
i) Tasks have ”thin context” or no context.
ii) Students need not recognize or use traditional notation for quadrants (such as I, II, III, IV).
iii) Coordinates are not limited to integers.
no
5
6.C.4
Base explanations/reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 6.NS.6, 6.NS.7
Base explanations/reasoning on a coordinate plane diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 6.NS.6, 6.NS.8
6.C.5
3,5,6
yes
3,4,5,6
yes
Also check out Student Achievement Partners Coherence Map.
HOT Questions
1. If I reflected the points , and - over the y-axis, what would my new coordinate points be? Which Quadrant would the reflected point be plotted in?
2. Kathy used a number line to subtract -3 from 5.
a) Write the expression to represent -3 subtracted from 5. 5 – (- 3)
b) Will the answer be positive or negative? Positive
c) What is the answer? 8
3. Use the vertical number line to subtract
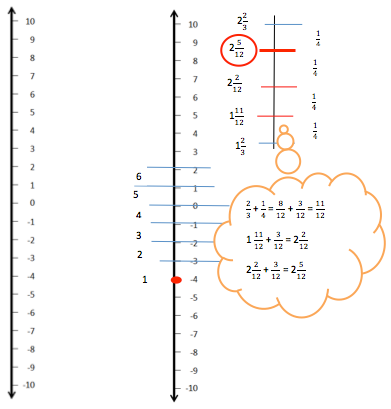
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/6/tasks/1665
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/6/tasks/1998
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/6/tasks/1999
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/6/tasks/2009
http://tasks.illustrativemathematics.org/content-standards/6/NS/C/6/tasks/2227
IL Classrooms in Action - Formative Assessment
http://www.mathteachersinaction.org/formative-assessment-tasks.html
Inside Mathematics – Performance Assessment Task
http://www.insidemathematics.org/assets/common-core-math-tasks/percent%20cards.pdf
EngageNY – 6.NS.6b
https://www.engageny.org/ccls-math/6ns6b
Howard County
https://grade6commoncoremath.wikispaces.hcpss.org/Unit+2+The+Number+System
Dana Center Task
http://ccsstoolbox.agilemind.com/parcc/about_middle_3794.html
