TEACHER GUIDE TO CLARIFICATION
Represent 3-D Figures using Nets and find Surface Area
A net is a two-dimensional representation of a three-dimensional figure.
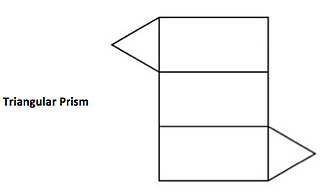

6.G.4 is limited to nets made up of rectangles and triangles.
Students represent three- dimensional figures whose nets are composed of rectangles and triangles. Students recognize that parallel lines on a net are congruent. Using the dimensions of the individual faces, students calculate the area of each rectangle and/or triangle and add these sums together to find the surface area of the figure.
It’s very important for students to physically manipulate materials and make connections to the symbolic and more abstract aspects of geometry. See Instructional Strategies G.1 for more information on exploring nets and making connections)
Instructional Strategies: 6.G.1
It is very important for students to continue to physically manipulate materials and make connections to the symbolic and more abstract aspects of geometry. Exploring possible nets should be done by taking apart (unfolding) three-dimensional objects. This process is also foundational for the study of surface area of prisms. Building upon the understanding that a net is the two-dimensional representation of the object, students can apply the concept of area to find surface area. The surface area of a prism is the sum of the areas for each face.
Multiple strategies can be used to aid in the skill of determining the area of simple two-dimensional composite shapes. A beginning strategy should be to use rectangles and triangles, building upon shapes for which they can already determine area to create composite shapes. This process will reinforce the concept that composite shapes are created by joining together other shapes, and that the total area of the two-dimensional composite shape is the sum of the areas of all the parts.

Fill prisms with cubes of different edge lengths (including fractional lengths) to explore the relationship between the length of the repeated measure and the number of units needed.
An essential understanding to this strategy is the volume of a rectangular prism does not change when the units used to measure the volume changes. Since focus in Grade 6 is to use fractional lengths to measure, if the same object is measured using one centimeter cubes and then measured using half centimeter cubes, the volume will appear to be eight times greater with the smaller unit. However, students need to understand that the value or the number of cubes is greater but the volume is the same.
Students construct models and nets of three dimensional figures, and describe them by the number of edges, vertices, and faces. Solids include rectangular and triangular prisms. Students are expected to use the net to calculate the surface area.
Students can create nets of 3D figures with specified dimensions using the Dynamic Paper Tool on NCTM’s Illuminations (http://illuminations.nctm.org/ActivityDetail.aspx?ID=205).
Students also describe the types of faces needed to create a three-dimensional figure. Students make and test conjectures by determining what is needed to create a specific three-dimensional figure.

Take a look at the questioning examples below. It is important to use questioning strategies that allow for students to determine the types of faces needed to create a 3D figure. Also, provide time for them to explore and try our their conjectures.
Examples:
* Describe the shapes of the faces needed to construct a rectangular pyramid. Cut out the shapes and create a model. Did your faces work? Why or why not?
* Create the net for a given prism or pyramid, and then use the net to calculate the surface area.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks. Questions or to send feedback: melisa@ksu.edu.
Retrieved from Math Flipbooks.
Example Problem
Retrieved from - http://www.shmoop.com/common-core-standards/ccss-6-g-4.html
Draw a net for the solid in Figure 2
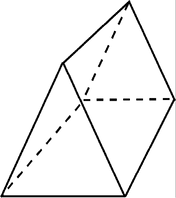
Figure 2
Answer net drawing for Figure 2

Dynamic Paper tool from Illuminations
Need a pentagonal pyramid that's six inches tall? Or a number line that goes from ‑18 to 32 by 5's? Or a set of pattern blocks where all shapes have one-inch sides? You can create all those things and more with the Dynamic Paper tool. Place the images you want, then export it as a PDF activity sheet for your students or as a JPG image for use in other applications or on the web.
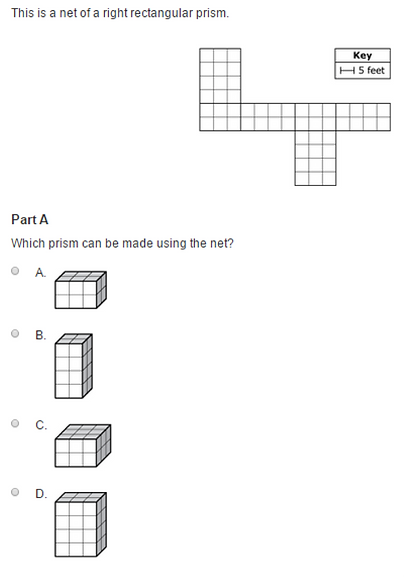

From the PARCC Grade 6 Mathematics EOY Computer Based Practice Test
http://parcc.pearson.com/practice-tests/math/
For additional information go to Achieve the Core.

6.G.4
Solve real-world and mathematical problems involving area, surface area, and volume.
6.G.4 Represent three-dimensional figures using nets made of rectangles and triangles, and use the nets to find the surface area of these figures. Apply these techniques in the context of solving real-world and mathematical problems.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
4.MD.3
5.NF.4
6.G.1
6.G.4
7.G.6
Examples of Opportunities for Connecting Mathematical Content and Mathematical Practices
Area, surface area, and volume present modeling opportunities (MP.4) and require students to attend to precision with the types of units involved (MP.6).
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Students also analyze and compose and decompose polyhedral solids. They describe the shapes of the faces, as well as the number of faces, edges, and vertices. They make and use drawings of solid shapes and learn that solid shapes have an outer surface as well as an interior. They develop visualization skills connected to their mathematical concepts as they recognize the existence of, and visualize, component of three-dimensional shapes that are not visible from a given viewpoint (MP.1). They measure the attributes of these shapes, allowing them to apply area formulas to solve surface area problems (MP.7). They solve problems that require them to distinguish between units used to measure volume and units used to measure area (or length). They learn to plan the construction of complex three-dimensional compositions through the creation of corresponding two-dimensional nets (e.g., through a process of digital fabrication and/or graph paper) 6.G.4. For example, they may design a living quarters (e.g., a space station) consistent with given specifications for surface area and volume (MP.2, MP.7).

Solve real-world and mathematical problems involving area, surface area, and volume.
Interesting to connect designing living quarters with specifications for surface area and volume. Be sure to include examples of real-world problems that include volume specifications and surface area.
In this and many other contexts, students learn to apply these strategies and formulas for areas and volumes to the solution of real-world and mathematical problems (6.G.1, 6.G.2). These problems include those in which areas and volumes are to be found from lengths or lengths are to be found from volumes or areas and lengths.
Common Core Standards Writing Team. (2012, June 23). Progressions for the Common Core State Standards in
Mathematics (draft). K-6 Geometry. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.
Illinois Assessment of Readiness Mathematics Evidence Statement Table
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
6.G.4
Represent three-dimensional figures using nets made up of rectangles and triangles, and use the nets to find the surface area of these figures. Apply these techniques in the context of solving real-world and mathematical problems.
1,4,5
yes
Calculator
Classroom Resources
Dynamic Paper tool from Illuminations
Need a pentagonal pyramid that's six inches tall? Or a number line that goes from ‑18 to 32 by 5's? Or a set of pattern blocks where all shapes have one-inch sides? You can create all those things and more with the Dynamic Paper tool. Place the images you want, then export it as a PDF activity sheet for your students or as a JPG image for use in other applications or on the web.
HOT Questions
1. I have created a toy box, it is a simple right rectangular prism. What is the surface area of the toy box?

Surface Area: 32 square feet.
2. The figure below is a triangular prism. Create a net and use the net to find the surface area of the pyramid.

Answer: net

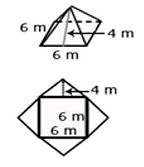
3. My friend Jax is making triangular prism blocks for his sister to play with. He creates nets and folds the blocks out
of cardstock. Below are the dimensions of the triangular blocks.
Jax plans to have at least 60 mm squared of cardstock for each block. Will this be enough?
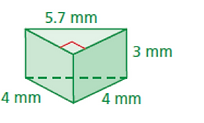
60mm squared of cardstock is not enough. The surface area of the triangular prism is 62.2mm squared.
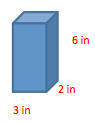
4. Find a rectangular prism with a surface area of 72 inches2.
Length = 3 in
Width = 2 in
Height = 6 in
5a. Draw the net of the shape with the dimensions labeled.

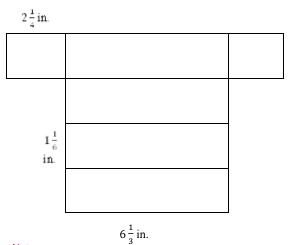
Nets may vary
5b. If you were going to wrap this box, how much wrapping paper would you need to buy?
A ≈ 31.36 in2 so at least 3ft by 3 ft to cover the box. A follow up question; Would gift wrap that comes 4ft by 2ft fit?
6. Camp Greenwood sells tents.

The area of the base is 64 square feet. The tent has a 7-foot zipper.
6a. Create a net for the given tent shown above.
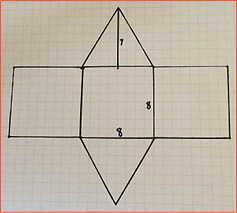
6.b Calculate the surface area of the tent.
SA = 248 units2
3(8x8) = 192 units2
2 (1/2(8x7))) = 56 units2
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/6/G/A/4/tasks/1985
The Teaching Channel
https://www.teachingchannel.org/videos/teaching-surface-area
Howard County
Also check out Student Achievement Partners Coherence Map.
