FIFTH GRADE > 5.NF.6 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION

5.NF.6
Apply and extend previous understandings of multiplication and division to multiply and divide fractions.
5.NF.6 Solve real world problems involving multiplication of fractions and mixed numbers, e.g., by using visual fraction models or equations to represent the problem.
Using Visual Fractional Models … to Represent Problems
Connect the meaning of multiplication and division of fractions with whole-number multiplication and division. Consider area models of multiplication and both sharing and measuring models for division.
Ask questions such as, “What does 2 × 3 mean?” and “What does 12 ÷ 3 mean?” Then, follow with questions for multiplication with fractions, such as, “What does 3/4 ×1/3 mean?” “What does 3/4 × 7 mean?” (7 sets of 3/4 ) and “What does 7 × 3/4 mean?”(3/4 of a set of 7)
The meaning of 4 ÷ 1/2 (how many 1/2 are in 4) and 1/2 ÷ 4 (how many groups of 4 are in 1/2) also should be illustrated with models or drawings like:
Encourage students to use models or drawings to multiply or divide with fractions. Begin with students modeling multiplication and division with whole numbers. Have them explain how they used the model or drawing to arrive at the solution.
Models to consider when multiplying or dividing fractions include, but are not limited to: area models using rectangles or squares, fraction strips/bars and sets of counters.
Present problem situations and have students use models and equations to solve the problem. It is important for students to develop understanding of multiplication and division of fractions through contextual situations.
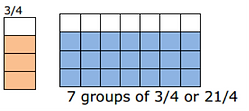
Example: There are 2 busloads of students standing in the parking lot. The students are getting ready to go on a field trip. of the students on each bus are girls. How many busses would it take to carry only the girls?
Student 1
I drew 3 grids and 1 grid represents 1 bus. I cut the third grid in half and I marked out the right half of the third grid, leaving 2 1⁄2 grids. I then cut each grid into fifths, and shaded two-fifths of each grid to represent the number of girls. When I added up the shaded pieces, 2/5 of the 1st and 2nd bus were both shaded, and 1/5 of the last bus was shaded.
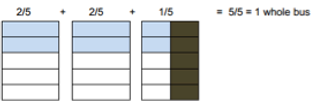
Student 2
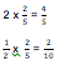
I split the 2 into 2 and
I then added and .
That equals 1 whole busload.
Example 2:
Evan bought 6 roses for his mother. of them were red. How many red roses were there?
Using a visual, a student divides the 6 roses into 3 groups and counts how many are in 2 of the 3 groups.
-
A student can use an equation to solve.
-
Mary and Joe determined that the dimensions of their school flag needed to be 1 ft. by 2 ft. What will be the area of the school flag?
-
A student can draw an array to find this product and can also use his or her understanding of decomposing numbers to explain the multiplication. Thinking ahead a student may decide to multiply by 1 instead of 2 .
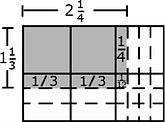
The explanation may include the following:
-
First, I am going to multiply 2 by 1 and then by .
-
When I multiply 2 by 1, it equals 2 .
-
Now I have to multiply 2 by .
-
times 2 is
-
times is
-
So the answer is
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.
Recommendation 3.
Help students understand why procedures for computations with fractions make sense.
Use area models, number lines, and other visual representations to improve students’ understanding of formal computational procedures.
Provide opportunities for students to use estimation to predict or judge the reasonableness of answers to problems involving computation with fractions.
Address common misconceptions regarding computational procedures with fractions.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
3.OA.1
3.OA.2
4.MD.2
5.NF.6
5.MD.2
5.NF.4
5.NF.4a
5.NF.5
5.NF.5a
5.NF.5b
5.NF.7
5.NF.7a
5.NF.7b
5.NF.7c
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
5.NF.6-1
Solve real world problems involving multiplication of fractions, e.g., by using visual fraction models or equations to represent the problem.
i). Tasks do not involve mixed numbers.
ii). Situations include area and comparison/times as much, with product unknown. (See Table 2, p. 89 of CCSS and Table 3, p. 23 of Progression for Operations and Algebraic Thinking.)
iii).Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy.
1,4,5
5.NF.6-2
Solve real world problems involving multiplication of fractions and mixed numbers, e.g., by using visual fraction models or equations to represent the problem.
i). Tasks present one or both factors in the form of a mixed number.
ii). Situations include area and comparison/times as much, with product unknown.
iii). Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy.
1,2,5
Illinois Assessment of Readiness Mathematics Evidence Tables.
Retrieved from: https://www.isbe.net/Documents/IAR-Grade-5-Math-Evidence-State.pdf
This content standard connects nicely to Math Practice Standard 6 ATTEND TO PRECISION
Students attend carefully to the underlying unit quantities when solving problems. For example, if 1/2 of a fund raiser's funds were raised by the 6th grade, and if 1/3 of the 6th grade's funds were raised by Ms. Wilkin's class, then 1/3 x 1/2 gives the fraction of the fund-raiser's funds that Ms. Wilkin's class raised, but it does not tell us how much money Ms. Wilkin's class raised.
Common Core Standards Writing Team. (2013, September 19).
Progressions for the Common Core State Standards in Mathematics(draft). 3-5 Number and Operations - Fractions. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.
These real world problems involving multiplication of fractions and mixed numbers should have a variety of problem solving situations see TABLE 2 PAGE 89 OF THE COMMON CORE STANDARDS
Also check out Student Achievement Partners Coherence Map.
Classroom Resources
The PowerPoint gives the students opportunities to solve real world problems by using visual fraction models or equations to represent the problem. It has both area and comparison situation types.
Fraction manipulative template
http://www.cehd.umn.edu/ci/rationalnumberproject/flash/circles.swf
Comparison Fraction Multiplication
Number Line
Equation
John sprinted 3/4 of the way around the track. His little brother Matt could only sprint 2/3 of that distance. How far could Matt sprint around the track?
Area Fraction Multiplication
Area Model
Equation
Josh was cutting the grass in the yard on Monday. He was half way done when it started to rain. The next day he was able to cut one-third of the remaining grass.
How much of the yard did Josh cut on Tuesday?
HOT Questions
For the pictures below write an equation and a story problem to match the picture. The shaded part of the model represents the answer.

Equation:
Story problem:
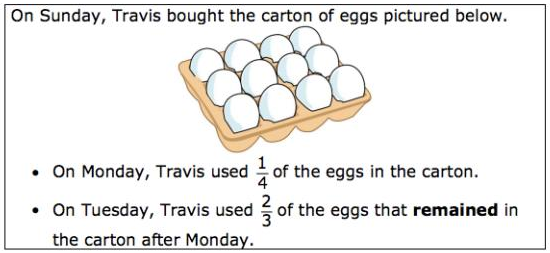

My answer is ________________________________________ because ___________________________________________
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/294
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/295
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/296
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/297
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/609
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/1174
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/2077
http://tasks.illustrativemathematics.org/content-standards/5/NF/B/6/tasks/2102
Howard County
https://hcpss.instructure.com/courses/108/pages/5-dot-nf-dot-6-about-the-math-learning-targets-and-rigor
IL Classrooms in Action - Formative Assessment
http://www.mathteachersinaction.org/formative-assessment-tasks.html

