5.NF.4 Teacher Guide | ilteachandtalk

FIFTH GRADE > 5.NF.4 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION

5.NF.4

VISUAL FRACTION MODELS & REPRESENT PRODUCTS AS RECTANGULAR AREAS

COHERENCE AND CONNECTIONS

CLASSROOM RESOURCE HOT QUESTIONS ADDITIONAL RESOURCES

5.NF.4

Apply and extend previous understandings of multiplication and division to multiply and divide fractions.

5.NF.4 Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.

5.NF.4a. Multiply Fractions and Interpret Products: Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4=8/3, and create a story context for this equation. Do the same with (2/3) × (4/5)=8/15. (In general, (a/b) × (c/d)=ac/bd.)

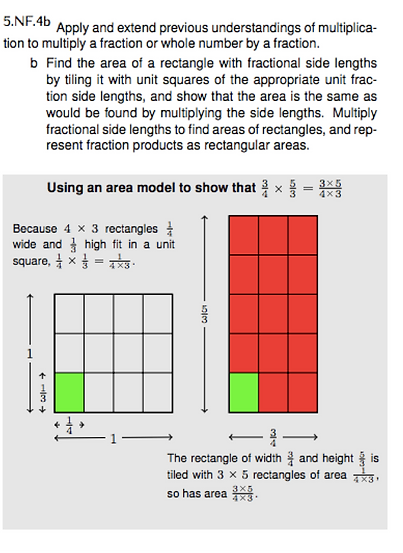
5.NF.4b. Find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.

Visual Fraction Models & Represent Products as Rectangular Areas

This standard is NOT about answering getting,

1. Multiply the top numbers (the numerators).

2. Multiply the bottom numbers (the denominators).

3. Simplify

Explanation and Examples:

This standard extends student’s work of multiplication from earlier grades. In fourth grade, students worked with recognizing that a fraction such as actually could be represented as 3 pieces that are each one-fifth ( ).

In fifth grade, students are expected to multiply fractions including proper fractions, improper fractions, and mixed numbers. They multiply fractions efficiently and accurately as well as solve problems in both contextual and non-contextual situations.

As they multiply fractions such as × 6, they can think of the operation in more than one-way.

Students create a story problem for × 6 such as, Isabel had 6 feet of wrapping paper. She used of the paper to wrap some presents. How much does she have left?

Kansas Association of Teachers of Mathematics (KATM) Flipbooks.

Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.

There are no standards regarding simplifying fractions. Instead teach students equivalent fractions.

Examples of Key Advances from Grade 4 to Grade 5

Students apply and extend their previous understanding of multiplication to multiply a fraction or whole number by a fraction (5.NF.4). They also learn the relationship between fractions and division, allowing them to divide any whole number by any nonzero whole number and express the answer in the form of a fraction or mixed number (5.NF.3). And they apply and extend their previous understanding of multiplication and division to divide a unit fraction by a whole number or a whole number by a unit fraction. Students able to multiply fractions in general can develop strategies to divide fractions in general by reasoning about the relationship between multiplication and division. But the division of a fraction by a fraction is not a requirement in this grade.

Examples of Major Within-Grade Dependencies

Understanding that in a multi-digit number, a digit in one place represents 1/10 of what it represents in the place to its left (5.NBT.1) is an example of multiplying a quantity by a fraction (5.NF.4).

Examples of Opportunities for In-Depth Focus

5.NF.4 When students meet this standard, they fully extend multiplication to fractions, making division of fractions in grade 6 (6.NS.1) a near target.

PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).

Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf

For additional information go to Achieve the Core.

Coherence and Connections: Need to Know

Grade Below

Grade Level

Grade Above

3.MD.7

4.NF.4

5.NF.4b

5.NF.3

5.NF.6

6.NS.1

5.NF.4 builds on previous understating of multiplication. Grade 5 students need not express multiplication of factions as procedural, but rather reason out the answer through repeated addition and concrete models such as fraction strips, number line diagrams and area models.

Common Core Standards Writing Team. (2013, September 19).

Progressions for the Common Core State Standards in Mathematics(draft). 3-5 Number and Operations - Fractions. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.

Evidence

Statement Key

Evidence Statement Text

Clarifications

5.C.2-3

Base explanations/reasoning on the relationship between multiplication and division.

Content Scope: Knowledge and skills articulated in 5.NF.3, 5.NF.4a

2,3,6,7

5.C.4-2

Base arithmetic explanations/reasoning on concrete referents such as diagrams (whether provided in the prompt or constructed by the student in her response), connecting the diagrams to a written (symbolic) method.

Content Scope: Knowledge and skills articulated in 5.NF.4b

None

2,3,5,6

5.C.5-2

Base explanations/reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response).

Content Scope: Knowledge and skills articulated in 5.NF.4a

None

3,6,7

5.NF.4a-1

Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.

Multiply Fractions and Interpret Products: Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4 = 8/3, and create a story context for this equation. Do the same with (2/3) × (4/5) = 8/15. (In general, (a/b) × (c/d) = ac/bd.)

i) Tasks require finding a fractional part of a whole number quantity

ii) The result is equal to a whole number 20% of the tasks

iii) Tasks have thin context or no context

None

5.NF.4a-2

Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.

i) Tasks have “thin context” or no context.

ii) Tasks require finding a product of two fractions (neither of the factors equal to a whole number).

iii) The result is equal to a whole number in 20% of tasks; these are practice-forward for MP.7.

5.NF.4b-1

Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.

b. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.

i) 50% of the tasks present students with the rectangle dimensions and ask students to find the area; 50% of the tasks give the factions and the product and ask students to show a rectangle to model the problem.

2,5

Illinois Assessment of Readiness Mathematics Evidence Tables.

Retrieved from: https://www.isbe.net/Documents/IAR-Grade-5-Math-Evidence-State.pdf

Base explanations/reasoning on the relationship between multiplication and division

Base arithmetic explanations/reasoning on concrete referents

Also check out Student Achievement Partners Coherence Map.

Classroom Resources

5.NF.4 Daily Discourse

Math Journal

x 6

Use the above equation to create a story problem. Solve the problem and show your work with a visual model.

http://practice.parcc.testnav.com/

HOT Questions

1. Which statement can be represented by the expression ?

a. 4 parts of a whole partitioned into 3 equal parts added 7 times

b. 7 parts of a whole partitioned into 3 equal parts added 4 times

c. 4 parts of a whole partitioned into 7 equal parts added 3 times

d. 3 parts of a whole partitioned into 4 equal parts added 7 times

2. Carol created a square-shaped poster. The length of each side of this poster is 2 ½ feet.

What is the area of this poster, in square feet? __________________________

Will created a rectangular poster with the same area as Carol’s poster. His poster has different dimensions than Carol’s poster. What could be the dimensions of Will’s poste, in feet?

Show your work with a visual model.

3. A rectangle is twice as long as it is wide. It’s width is 6 feet. Find the area of the rectangle.

4. Create a story and equation to match this visual model

5. Katrina’s closet is 5 feet long and 3 feet wide. Below is an area model of where she would like to place the rug in her closet.

Prove that if Katrina counts the tiles to find the area of the rug she will get the same answer as she gets by multiplying the length and width of the rug.

6. Ben is calculating the area of his desk that is 3 by 5 feet long.

He said he could solve it with the expression (3 X 5) +

Do you agree with Ben’s thinking? Justify your answer with both words and a visual model.