FIFTH GRADE > 5.NBT.6 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION

5.NBT.6
Perform operations with multi-digit whole numbers and with decimals to hundredths.
5.NBT.6
Find whole-number quotients of whole numbers with up to four-digit dividends and two-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
Quotients Based on Strategies
Explanation: In 4th Grade, students' experience with division were limited to dividing by one-digit divisors. This standard extends students' prior experiences with strategies, illustrations, and explanations. When the two-digit divisor is a "familiar" number, a student might decompose the dividend using place value.
Examples:
-
Using expanded notation 2682 ÷ 25=(2000 + 600 + 80 + 2) ÷ 25
-
Using his or her understanding of the relationship between 100 and 25, a student might think:
-
I know that 100 divided by 25 is 4, so 200 divided by 25 is 8, and 2000 divided by 25 is 80.
-
600 divided by 25 has to be 24.
-
Since 3 x 25 = 75, I know that 80 divided by 25 is 3 with a remainder of 5. (Note that a student might divided into 82 and not 80).
-
I cannot divide 2 by 25 so 2 plus 5 leaves a remainder of 7.
-
80 + 24 + 3 = 107 so the answer is 107 with a remainder of 7.
-
Using an equation that relates division to multiplication, 25 x n = 2682, a student might estimate the answer to be slightly larger than 100 because he or she recognizes that 25 x 100 = 2500.
-
968 ÷ 21
-
Using base ten models, a student can represent 966 and use the models to make an array with one dimension of 21. The student continues to make the array until no more groups of 21 can be made. Remainders are not part of the array.
-
So, 210 x 4 = 840
21 x 6 = 126
966
+ 2 remainder
So, (21 x 46) + 2 = 968
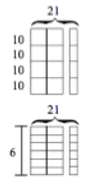

Note how equations are used based on the drawing or model. Students need to develop the ability to create equations from their drawings or models.
-
9984 ÷ 64
-
An area model for division is shown below. As students use the area model, they keep track of how much of the 9984 is left to divide.
-
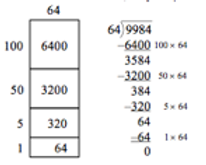
So, 9984 ÷ 64 = 156.
Sample Problems:
David does the same number of pulls-ups each day. If he did 3,813 pull-ups in 31 days, how many pulls-up did he do each day?
Relationship Between Multiplication and Division
Catherine solved the problem, 3,813 ÷ 31=123, and thought her answer was incorrect.
What multiplication equation can she use to check her work?
3,813 = 31 x 123
Area Model
3,813 ÷ 31 = 123
100 + 20 + 3
31
3100
620
93
For additional information go to Achieve the Core.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
4.NBT.4
4.NBT.6
5.NBT.65.NBT.1 5.NBT.55.NBT.7
6.NS.26.NS.3
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
5.C.1.1
Base explanations/reasoning on the properties of operations. Content Scope: Knowledge and skills articulated in 5.NBT.6
i) Students need not use technical terms such as commutative, associative, distributive, or property.
ii) Tasks do not have a context.
3,7,5,6
5.C.2.1
Base explanation/reasoning on the relationship between addition and subtraction or the relationship between multiplication and division.
Content Scope: Knowledge and skills articulated in 5.NTB.6
None
3,7,5,6
5.C.4.3
Base arithmetic explanations/reasoning on concrete referents such as diagrams (whether provided in the prompt or constructed by the student in her response), connecting the diagrams to a written (symbolic) method.
Content Scope: Knowledge and skills articulated in 5.NBT.6
None
3,5,6
5.NBT.6
Find whole-number quotients of whole numbers with up to four-digit dividends and two-digit divisors, using strategies based on place value, the properties of operations, and/or the relationship between multiplication and division. Illustrate and explain the calculation by using equations, rectangular arrays, and/or area models.
i) Tasks do not require students to illustrate or explain.
ii) Tasks involve 3- or 4-digit dividends and one- or two-digit divisors.
1,5
Illinois Assessment of Readiness Mathematics Evidence Tables.
Retrieved from: https://www.isbe.net/Documents/IAR-Grade-5-Math-Evidence-State.pdf
Examples of Opportunities for In-Depth Focus
5.NBT.6 The extension from one-digit divisors to two-digit divisors requires care. This is a major milestone along the way to reaching fluency with the standard algorithm in grade 6 (6.NS.2).
Examples of Opportunities for Connecting Mathematical Content and Mathematical Practices
Mathematical practices should be evident throughout mathematics instruction and connected to all of the content areas highlighted above, as well as all other content areas addressed at this grade level. Mathematical tasks (short, long, scaffolded, and unscaffolded) are an important opportunity to connect content and practices. Some brief examples of how the content of this grade might be connected to the practices follow. When students break divisors and dividends into sums of multiples of base-ten units (5.NBT.6), they are seeing and making use of structure (MP.7) and attending to precision (MP.6). Initially for most students, multidigit division problems take time and effort, so they also require perseverance (MP.1) and looking for and expressing regularity in repeated reasoning (MP.8).
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Also check out Student Achievement Partners Coherence Map.
Classroom Resources
ISBE Model Math Curriculum Grade 5 Unit 1
At Maria’s school, 6 classes are going on a field trip. Each class has 26 students and 1 teacher. Each bus holds a maximum of 48 people. The school requests 3 buses for the field trip. Read Maria’s argument below. Circle the statement in Maria’s argument that has incorrect reasoning or inaccurate calculations. Then correct the statement.
a. Maria says that 3 buses are not enough.
b. She argues that 3 buses will hold a maximum of 144 people.
c. The classes need space for 156 people.
d. The school needs to order 1 more bus.
Resource: Smarter Balanced Assessment Consortium Practice test Grade 5, 2013
HOT Questions
Each member of Mark's school band sold the same number of tickets to their concert. Altogether the members of the school band sold a total of 442 tickets. There are 34 members of the band. To determine the number of tickets each member sold, Mark used the model shown.
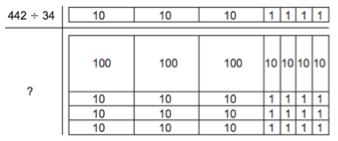
How many tickets did each member of Mark’s school band sell?
http://www.louisianabelieves.com/docs/assessment/practice-test-math-grade-5.pdf?sfvrsn=4
Use the digits 1, 2, 5, 7 and 9 each once to create the largest quotient
Deb solved 585 ÷ 45 with this area model but she doesn’t know the solution. Explain to her how to get the answer form her work.
10 2 1
45
450
90
45
585 135 45
-450 -90 -45
------ ----- ----
Select all the equations that will solve 4525 ÷ 25. Let equal the quotient.
A. n ÷ 25 = 4525
B. n ÷ 4535 = 25
C. 4525 ÷ 25 = n
D. 25 ÷ 4525 = n
E. 25 x 4525 = n
F. 4525 = n x 25
G. 25 x n = 4525
H. 4525 = n
25
Additional Resources
PARCC sample EOY TEST
http://practice.parcc.testnav.com/
Number 27
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/5/NBT/B/6/tasks/878
Howard County
K-5 Teaching Resources
https://www.k-5mathteachingresources.com/5th-grade-number-activities.html
Learnzillion
https://learnzillion.com/lessons/551

