4.NF.4 Teacher Guide | ilteachandtalk

FOURTH GRADE > 4.NF.4 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION

4.NF.4 UNIT FRACTIONS AND FRACTION MODELS

COHERENCE AND CONNECTIONS

CLASSROOM RESOURCE HOT QUESTIONS ADDITIONAL RESOURCES

4.NF.4

Build fractions from unit fractions by applying and extending previous understandings of operations on whole numbers.

4.NF.4 Apply and extend previous understanding of multiplication to multiply a fraction by a whole number.

Understand a fraction a/b as a multiple of 1/b. For example, use a visual fraction model to represent 5/4 as the product of 5 x (1/4), recording the conclusion by the equation 5/4=5 x (1/4).
Understand a multiple of a/b as a multiple of 1/b, and use this understanding to multiply a fraction by a whole number. For example, use a visual fraction model to express 3 x (2/5) as 6 x (1/5), recognizing this product as 6/5. (in general, n x (a/b)=(n x a )/b).
Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?

Note: Grade 4 expectations in this domain are limited to fractions with denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100.

Unit Fractions and Fraction Models

Students need many opportunities to work with problems in context to understand the connections between models and corresponding equations. Contexts involving a whole number times a fraction lend themselves to modeling and examining patterns. This standard builds on students’ work of adding fractions and extending that work into multiplication. (4.NF.4a)

Example:

If each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?

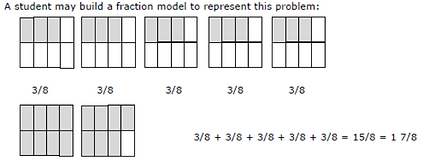
This standard extends the idea of multiplication as repeated addition (4.NF.4b).

For example, 3 x (2/5)=2/5 + 2/5 + 2/5=6/5=6 X (1/5). Students are expected to use and create visual fraction models to multiply a whole number by a fraction.

This standard calls for students to use visual fraction models (Area, Linear and Set Models) to solve word problems related to multiplying a whole number by a fraction. (4.NF.4c)

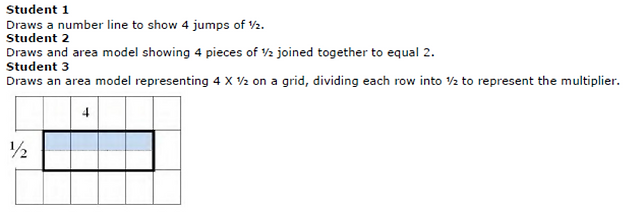
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.

Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.

For additional information go to Achieve the Core.

Coherence and Connections: Need to Know

Grade Below

Grade Level

Grade Above

3.OA.1

3.NF.1

4.OA.2

4.NF.4

4.MD.2

5.NF.4a

5.NF.7

Previously, in Grade 3, students learned that 3 x 7 can be represented as the number of objects in 3 groups of 7 objects, and write this as 7 + 7 + 7. Grade 4 students apply this understanding to fractions, seeing 1/3 +1/3 +1/3 +1/3 + 1/3 as 5 x 1/3.

In general, they see a fraction as the numerator times the unit fraction with the same denominator (4.NF.4a), e.g.,

The same thinking, based on the analogy between fractions and whole numbers, allows students to give meaning to the product of a whole number and a fraction, (4.NF.4b) e.g., they see

Students solve word problems involving multiplication of a fraction by a whole number (4.NF.4c).

Common Core Standards Writing Team. (2013, September 19).

Progressions for the Common Core State Standards in Mathematics(draft). K-5 Counting and Cardinality and Operations and Algebraic Thinking. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.

This standard, 4.NF.4, represents an important step in the multi-grade progression for multiplication and division of fractions. Students extend their developing understanding of multiplication to multiply a fraction by a whole number.

The work that students do with units of measure (4.MD.1-2) and with multiplication of a fraction by a whole number (4.NF.4) can be connected to the idea of “times as much” in multiplication (4.OA.1).

Standard 4.MD.2 refers to using the four operations to solve word problems involving measurement quantities such as liquid volume, mass, time, and so on. Some parts of this standard could be met earlier in the year (such as using whole-number multiplication to express measurements given in a larger unit in terms of a smaller unit — see also 4.MD.1), while others might be met only by the end of the year (such as word problems involving addition and subtraction of fractions or multiplication of a fraction by a whole number — see also 4.NF.3d and 4.NF.4c).

PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).

Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf.

Evidence

Statement Key

Evidence Statement Text

Clarifications

4.NF.4b-1

Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.

b. Understand a multiple a/b as a multiple of 1/b. For example, use a visual fraction model to express

3 x 2/5=6 x 1/5.

4.NF.4b-2

Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.

b. Use the understanding that a multiple of a/b is a multiple of 1/b to multiply a fraction by a whole number.

For example, use a visual fraction model to express

3x 2/5 as 6/5 (In general,

i) Tasks do not have a context.

ii) Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy.

iii) Tasks involve expressing a multiple of a/b as a fraction.

iv) Results may equal fractions greater than 1 (including those equal to whole numbers).

v) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100.

7,5

Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.

c. Solve word problems involving multiplication of a fraction by a whole number, e.g., by using visual fraction models and equations to represent the problem. For example, if each person at a party will eat 3/8 of a pound of roast beef, and there will be 5 people at the party, how

many pounds of roast beef will be needed? Between what two whole numbers does your answer lie?

i) Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy.

ii) Situations are limited to those in which the product is unknown

(situations do not include unknown factors).

iii) Situations involve a whole number of fractional quantities—not a fraction of a whole-number quantity.

iv) Results may equal fractions greater than 1 (including fractions equal to whole numbers).

v) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

1, 4, 5

4.NF.4c

4.C.4-3

Base arithmetic explanations/reasoning on concrete referents such as diagrams (whether provided in the prompt or constructed by the student in her response), connecting the diagrams to a written (symbolic) method.

Content Scope: Knowledge and skills articulated in 4.NF.4a

i) Tasks have “thin context” or no context.

ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

3, 5, 6

4.C.4-4

i) Tasks have “thin context” or no context.

ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

2, 3, 5, 6

i) Tasks have “thin context” or no context.

ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

Content Scope: Knowledge and skills articulated in 4.NF.4b

Base arithmetic explanations/ reasoning on concrete referents such as diagrams (whether provided in the prompt or constructed by the student in her response), connecting the diagrams to a written (symbolic) method.

Content Scope: Knowledge and skills articulated in 4.NF.C

4.C.4-5

4.C.5-4

Distinguish correct explanation/reasoning from that which is flawed, and – if there is a flaw in the argument – present corrected reasoning. (For example, some flawed “student” reasoning is presented and the task is to correct and improve it.) Content Scope: Knowledge and skills articulated in 4.NF.B

i) Grade 4 expectations in NF are limited to fractions with denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p.

30).

ii) For fractions equal to a whole number, values are limited to 0,

1, 2, 3, 4, and 5.

3, 5, 6

4.C.6-3

Present solutions to multi-step problems in the form of valid chains of reasoning, using symbols such as equals signs appropriately (for example, rubrics award less than full credit for the presence of nonsense statements such as

1+4=5+7=12, even if the final answer is correct), or identify or describe errors in solutions to multi-step problems and present corrected solutions. Content Scope: Knowledge and skills articulated in 4.NF.3d, 4.NF.4c

i) Denominators are limited to grade 3 possibilities (2, 3, 4, 6, 8)

so as to keep computational difficulty lower (CCSS footnote, p. 24).

3,6,2,5

4.C.7-4

Base explanations/ reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response).

Content Scope: Knowledge and skills articulated in

4.NF.4a, 4.NF.4b

None

3,5,6

Apply and extend previous understandings of multiplication to multiply a fraction by a whole number.

a. Understand a fraction a/b as a multiple of 1/b.

For example, use a visual fraction model to represent 5/4 as

the product 5 x 1/4 recording the conclusion by the equation

5/4=5 x 1/4.

i) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

4.NF.4a

5,7

4.NF.Int.1

i) See ITN Appendix F, section A, “Illustrations of Innovative

Task Characteristics,” subsection 4, “Integrative tasks with machine scoring of responses entered by computer interface.”

ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

1,4

Express a fraction with denominator 10 as an equivalent fraction with denominator 100, and use this technique to add two fractions with respective denominators 10 and 100. For example, express 3/10 as 30/100, and add

4.NF.Int.2

i) Tasks are one-step addition word problems of one of two kinds: Add To with result unknown, or Put Together with result unknown.

ii) See Table 2, p. 9 of the Progression for Operations and

Algebraic Thinking; these situations are sampled equally.

i) Tasks do not have a context.

ii) Prompts do not provide visual fraction models; students may at

their discretion draw visual fraction models as a strategy.

iii) Tasks involve expressing a multiple of a b/ as a multiple of

1/b.

iv) Results may equal fractions greater than 1 (including those

equal to whole numbers).

v) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).

Solve one-step word problems requiring integration of

knowledge and skills articulated in 4.NF

7,5

Illinois Assessment of Readiness Mathematics Evidence Tables.

Retrieved from: https://www.isbe.net/Documents/IAR-Grade-4-Math-Evidence-State.pdf.

Also check out Student Achievement Partners Coherence Map.

Classroom Resources

4.NF.4 Daily Discourse

Free Printable Fraction Cards
http://www.teacherspayteachers.com/Product/FREE-Fraction-Cards-for-sorting-matching-other-hands-on-activities-497377

HOT Questions

1. Carla said 4 x3/7 is the same as 12 x 1/7. Do you agree or disagree? Explain your reasoning. Draw visual fraction models as part of your justification.

2. What two factors can be multiplied to equal a product of 9/12? How many can you come up with?

3. Write two equivalent equations to 3 x 6/9.