i) Tasks do not have a context.
ii) Prompts do not provide visual fraction models; students may at
their discretion draw visual fraction models as a strategy.
iii) Tasks involve expressing a multiple of a b/ as a multiple of
1/b.
iv) Results may equal fractions greater than 1 (including those
equal to whole numbers).
v) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
FOURTH GRADE > 4.NF.3 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION

4.NF.3
Build fractions from unit fractions by applying and extending previous understandings of operations on whole numbers.
4.NF.3 Understand a fraction a/b with a>1 as a sum of fractions 1/b.
-
Understand addition and subtraction of fractions as joining and separating parts referring to the same whole.
-
Decompose a fraction into a sum of fractions with the same denominator in more than one way, recording each decomposition by an equation. Justify decompositions, e.g., by using a visual fraction model.
Examples: 3/8=1/8 + 1/8 + 1/8; 3/8=1/8 + 2/8;
2 1/8=1 +1 + 1/8=8/8 + 8/8 + 1/8.
-
Add and subtract mixed numbers with like denominators, e.g., by replacing each mixed number with an equivalent fraction, and/or by using properties of operations and the relationship between addition and subtraction.
-
Solve word problems involving addition and subtraction of fractions referring to the same whole and having like denominators, e.g., by using visual fraction models and equations to represent the problem.
Note: Grade 4 expectations in this domain are limited to fractions with denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100.
Unit Fractions and Fraction Models
A fraction with a numerator of one is called a unit fraction. When students investigate fractions other than unit fractions, such as 2/3, they should be able to decompose the non-unit fraction into a combination of several unit fractions.
Example: 2/3=1/3 + 1/3
Being able to visualize this decomposition into unit fractions helps students when adding or subtracting fractions. Students need multiple opportunities to work with mixed numbers and be able to decompose them in more than one way. Students may use visual models to help develop this understanding.
Example:
* 1 ¼ - ¾=
* 4/4 + ¼=5/4
* 5/4 – ¾=2/4 or ½
Example of word problem:
Mary and Lacey decide to share a pizza. Mary ate 3/6 and Lacey ate 2/6 of the pizza. How much of the pizza did the girls eat together?

Solution: The amount of pizza Mary ate can be thought of a 3/6 or 1/6 and 1/6 and 1/6. The amount of pizza Lacey ate can be thought of a 1/6 and 1/6. The total amount of pizza they ate is 1/6 + 1/6 + 1/6 + 1/6 + 1/6 or 5/6 of the whole pizza.
A separate algorithm for mixed numbers in addition and subtraction is not necessary. Students will tend to add or subtract the whole numbers first and then work with the fractions using the same strategies they have applied to problems that contained only fractions.
Example:
Susan and Avery need 8 3/8 feet of ribbon to package gift baskets. Susan has 3 1/8 feet of ribbon and Avery has 5 3/8 feet of ribbon. How much ribbon do they have altogether? Will it be enough to complete the project? Explain why or why not.
The student thinks: I can add the ribbon Susan has to the ribbon Avery has to find out how much ribbon they have altogether. Susan has 3 1/8 feet of ribbon and Avery has 5 3/8 feet of ribbon. I can write this as 3 1/8 + 5 3/8. I know they have 8 feet of ribbon by adding the 3 and 5. They also have 1/8 and 3/8 which makes a total of 4/8 more. Altogether they have 8 4/8 feet of ribbon. 8 4/8 is larger than 8 3/8 so they will have enough ribbon to complete the project. They will even have a little extra ribbon left, 1/8 foot.
Example:
Timothy has 4 1/8 pizzas left over from his soccer party. After giving some pizza to his friend, he has 2 4/8 of a pizza left. How much pizza did Timothy give to his friend?
Solution: Timothy had 4 1/8 pizzas to start. This is 33/8 of a pizza. The x’s show the pizza he has left which is 2 4/8 pizzas or 20/8 pizzas. The shaded rectangles without the x’s are the pizza he gave to his friend which is 13/8 or 1 5/8 pizzas.

Mixed numbers are introduced for the first time in Fourth Grade. Students should have ample experiences of adding and subtracting mixed numbers where they work with mixed numbers or convert mixed numbers into improper fractions.
Keep in mind Concrete-Representation-Abstract (CRA) approach to teaching fractions. Students need to be able to “show” their thinking using concrete and/or representations BEFORE they move to abstract thinking.
Example:
While solving the problem 3 ¾=2 ¼’ students could do the following:

Example:

Instructional Strategies
In Grade 3, students added unit fractions with the same denominator. Now, they begin to represent a fraction by decomposing the fraction as the sum of unit fraction and justify with a fraction model. For example, 3/4=1/4 + 1/4 + 1/4.

Students also represented whole numbers as fractions. They use this knowledge to add and subtract mixed numbers with like denominators using properties of number and appropriate fraction models. It is important to stress that whichever model is used, it should be the same for the same whole. For example, a circular model and a rectangular model should not be used in the same problem.
Understanding of multiplication of whole numbers is extended to multiplying a fraction by a whole number. Allow students to use fraction models and drawing to show their understanding.
Present word problems involving multiplication of a fraction by a whole number. Have students solve the problems using visual models and write equations to represent the problems.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from: Math Flipbooks
Representations for Concepts Before Procedure
* Before teaching students how to add with like denominators, have students initially use manipulatives. After they are comfortable with the concrete model, have them move onto the semi-concrete and draw pictures to show the addition and subtraction of fractions.
* Use fraction strips or fraction pieces to model addition of fractions with like denominators. Help students estimate a reasonable sum.
Good Questions / Problems about Fractions
Be sure to have students work with partners or small groups to write their answers to the questions below. It is critical that students have an opportunity to share their thoughts with the whole class after completing the problem.
* How do you know that 5/8 + 5/8 is more than one whole? Explain your thinking.
* Is 1 1/8 a reasonable answer for 3/8 + 2/8? Explain your thinking.
* If I subtract 3/8 from 7/8, is 4/16 a reasonable answer? Explain your thinking
Pattern Block Fractions (addition)

-
Ask students to predict what the sum of 4 1/3 + 2 2/3 is.
-
Using think-pair-share, have students describe why their predictions are correct.
-
Using pattern blocks (yellow hexagons and blue rhombi) model the two. Have students draw pictures of the models and record the sum. Make connections between the models and the numbers.
-
Have students create other addition sentences with mixed numbers using pattern blocks. Yellow hexagons should represent 1 whole. Red trapezoids should represent 1/2. Green triangles should represent 1/6.
-
Have students share their equations with the class.
Pattern Block Fractions (subtraction)
-
Ask students to predict what the difference of 2 4/6 - 3/6 is.
-
Using think-pair-share have students describe why their predictions are correct.
-
Using pattern blocks (yellow hexagons and blue rhombi) model the two. Have students draw pictures of the models and record the sum. Make connections between the models and the numbers.
-
Have students create other subtraction sentences with mixed numbers using pattern blocks. Yellow hexagons should represent 1 whole. Red trapezoids should represent 1/2. Green triangles should represent 1/6.
Teaching Student-Centered Mathematics (Grades 3-5)
pg. 150 * First Estimates Activity 5.13

Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
1.OA.3
1.OA.4
1.OA.8
2.OA.1
3.NF.1
3.NF.2
4.NF.1
4.NF.3
4.NF.5
4.MD.2
4.MD.4
5.NF.1
The meaning of addition is the same for both fractions and whole numbers, even though algorithms for calculating their sums can be different. Just as the sum of 4 and 7 can be seen as the length of segment obtained by joining together two segments of lengths 4 and 7, so the sum of 2/3 and 8/5 can be seen as the length of the segment obtained joining together two segments of lengths 2/3 and 8/5. It is not necessary to know how much 2/3 + 8/5 is exactly in order to know what the sum means. This is analogous to understanding 51 x 78 as 51 groups of 78, without necessarily knowing its exact value.

This simple understanding of addition as putting together allows students to see in a new light the way fractions are built up from unit fractions. The same representation students used in Grade 4 to see a fraction as a point on the number line now allows them to see a fraction as a sum of unit fractions: just as 5=1 + 1 + 1 + 1 + 1, so 5/3=1/3 + 1/3 + 1/3 + 1/3 + 1/3 because 5/3 is the total length of 5 copies of 1/3. (4.NF.3)

Armed with this insight, students decompose and compose fractions with the same denominator. They add fractions with the same denominator (4.NF.3c):

Using the understanding gained from work with the whole numbers of the relationship between addition and subtraction, they also subtract fractions with the same denominator. For example, to subtract 5/6 from 17/6, they decompose

Students also compose sums of whole numbers and fractions, by representing the whole number as an equivalent fraction with the same denominator as the fraction, e.g.,
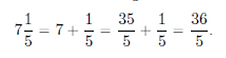
Students use this method to add mixed numbers with like denominators. Converting a mixed number to a fraction should not be viewed as a separate technique to be learned by rote, but simply as a case of fraction addition.
Changing mixed numbers to fractions is not a lesson. It is fraction addition and should ONLY be discussed this way.

Changing mixed numbers to fractions is not a lesson. It is fraction addition and should ONLY be discussed this way.
Similarly, converting an improper fraction to a mixed number is a matter of decomposing the fraction into a sum of a whole number and a number less than 1 (4.NF.3b). Students can draw on their knowledge from Grade 3 of whole numbers as fractions. For example, knowing that 1=3/3, they see
Repeated reasoning with examples that gain in complexity leads to a general method involving Grade 4 NBT skill of finding quotients and remainders (4.NBT.6). For example
When solving word problems, students learn to attend carefully to the underlying unit quantities. In order to formulate an equation of the form A + B=C or A – B=C for a word problem, the numbers A, B, and C must all refer to the same (or equivalent) wholes or unit amounts (4.NF.3d). For example, students understand that the problem “Bill had 2/3 of a cup of juice. He drank ½ of his juice. How much juice did Bill have left?” cannot be solved by subtracting 2/3 – ½ because the 2/3 refers to a cup of juice, but the ½ refers to the amount of juice that Bill had, and not to a cup of juice. Similarly, in solving “if ¼ of a garden is planted with daffodils, 1/3 with tulips, and the rest with vegetables, what fraction of the garden is planted with flowers?” students understand that the sum 1/3 + ¼ tells them the fraction of the garden that was planted with flowers, but not the number of flowers that were planted.
Common Core Standards Writing Team. (2013, September 19).
Progressions for the Common Core State Standards in Mathematics(draft). K-5 Counting and Cardinality and Operations and Algebraic Thinking. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.
This standard, 4.NF.3, represents an important step in the multi-grade progression for addition and subtraction of fractions. Students extend their prior understanding of addition and subtraction to add and subtract fractions with like denominators by thinking of adding or subtracting so many unit fractions.
Standard 4.MD.2 refers to using the four operations to solve word problems involving measurement quantities such as liquid volume, mass, time, and so on. Some parts of this standard could be met earlier in the year (such as using whole-number multiplication to express measurements given in a larger unit in terms of a smaller unit — see also 4.MD.1), while others might be met only by the end of the year (such as word problems involving addition and subtraction of fractions or multiplication of a fraction by a whole number — see also 4.NF.3d and 4.NF.4c).
Addition of fractions (4.NF.3) and concepts of angle measure (4.MD.5a and 4.MD.7) are connected in that a one-degree measure is a fraction of an entire rotation and that adding angle measures together is adding fractions with a denominator of 360.
PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).
Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf
Classroom Resources
Free Printable Fraction Cards
http://www.teacherspayteachers.com/Product/FREE-Fraction-Cards-for-sorting-matching-other-hands-on-activities-497377
HOT Questions
1. How many ways can you write 13/8 as a sum of fractions? Show your work.
2. Payton said that 1/5 + 1/5 is 2/10. Eli said 1/5 + 1/5=2/5. Who is correct? Why? Justify your answer with a visual fraction model.
3. If the sum of two mixed numbers is 8, what could the two addends be?
4. Is 4 5/7 + 2 3/7 greater than 8? How do you know? Justify your answer with a visual fraction model.
5. Write a word problem that has a sum of 3 ¼. Write a word problem that has a difference of 9 2/3.
Additional Resources
Illustrative Mathematics
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/831
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/837
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/835
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/968
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/856
http://tasks.illustrativemathematics.org/content-standards/4/NF/B/3/tasks/874
Howard County
IL Classrooms in Action - Formative Assessment
http://www.mathteachersinaction.org/formative-assessment-tasks.html
Georgia Department of Education
https://www.georgiastandards.org/Georgia-Standards/Frameworks/4th-Math-Unit-4.pdf
Evidence Table:
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
4.NF.3a
Understand a fraction a b/ with a > 1 as a sum of fractions
1/ b.
a. Understand addition and subtraction of fractions as joining and separating parts referring to the same whole.
4.NF.3b-1
Understand a fraction a b/ with a > 1 as a sum of fractions 1/ b.
b. Decompose a fraction into a sum of fractions with the same denominator in more than one way, recording each decomposition by an equation. Examples:
i) Only the answer is required (methods, representation, etc. are not assessed here); the justification aspect of 4.NF.3b is not assessed here.
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
iii) Tasks may include fractions that equal whole numbers.
7,8
Understand a fraction a b/ with a > 1 as a sum of fractions 1/ b.
d. Solve word problems involving addition and subtraction of fractions referring to the same whole and having like
denominators, e.g., by using visual fraction models and equations to represent the problem.
i) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
ii) Addition and subtraction situations are limited to the dark- or medium-shaded types in Table 2, p. 9 of the Progression for
Operations and Algebraic Thinking; these situations are sampled equally.
iii) Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy.
1, 4, 5
4.NF.3d
4.C.4-2
Base arithmetic explanations/reasoning on concrete referents such as diagrams (whether provided in the prompt or constructed by the student in her response), connecting the diagrams to a written (symbolic) method.
Content Scope: Knowledge and skills articulated in 4.NF.3a, 4.NF.3b
i) Tasks have “thin context” or no context.
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30)
3, 5, 6
4.C.5-4
i) Grade 4 expectations in NF are limited to fractions with denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p.
30).
ii) For fractions equal to a whole number, values are limited to 0,
1, 2, 3, 4, and 5.
3,6,5
2,3,6,7
i) Tasks have “thin context” or no context.
ii) Denominators are limited to grade 3 possibilities (2, 3, 4, 6, 8) so as to keep computational difficulty lower (CCSS footnote, p. 24).
Distinguish correct explanation/reasoning from that which is flawed, and – if there is a flaw in the argument – present corrected reasoning. (For example, some flawed “student” reasoning is presented and the task is to correct and improve it.) Content Scope: Knowledge and skills articulated in 4.NF.B
Present solutions to multi-step problems in the form of valid chains of reasoning, using symbols such as equals signs appropriately (for example, rubrics award less than full credit for the presence of nonsense statements such as
1+4=5+7=12, even if the final answer is correct), or identify or describe errors in solutions to multi-step problems and present corrected solutions. Content Scope: Knowledge and skills articulated in 4.NF.3c
4.C.6-2
4.C.6-3
Present solutions to multi-step problems in the form of valid chains of reasoning, using symbols such as equals signs appropriately (for example, rubrics award less than full credit for the presence of nonsense statements such as
1+4=5+7=12, even if the final answer is correct), or identify or describe errors in solutions to multi-step problems and present corrected solutions. Content Scope: Knowledge and skills articulated in
4.NF.3d, 4.NF.4c
i) Denominators are limited to grade 3 possibilities (2, 3, 4, 6, 8) so as to keep computational difficulty lower (CCSS footnote, p. 24).
3,6,2,5
4.C.7-3
Base explanations/ reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response).
Content Scope: Knowledge and skills articulated in 4.NF.3a
None
3,5,6
4.NF.3c
Understand a fraction a b/ with a > 1 as a sum of fractions 1/ b.
c. Add and subtract mixed numbers with like denominators, e.g., by replacing each mixed number with an equivalent fraction, and/or by using properties of operations and the relationship between addition and subtraction.
i) Tasks do not have a context.
ii) Denominators are limited to grade 3 possibilities (2, 3, 4, 6, 8) so as to keep computational difficulty lower (CCSS footnote, p. 24).
7
Solve one-step word problems requiring integration of
knowledge and skills articulated in 4.NF
i) See ITN Appendix F, section A, “Illustrations of Innovative
Task Characteristics,” subsection 4, “Integrative tasks with machine scoring of responses entered by computer interface.”
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
4.NF.Int.1
1,4
4.NF.Int.2
i) Tasks are one-step addition word problems of one of two kinds: Add To with result unknown, or Put Together with result unknown.
ii) See Table 2, p. 9 of the Progression for Operations and
Algebraic Thinking; these situations are sampled equally.
1
1,2,5
Solve real-world and mathematical problems about perimeter involving grade-level addition and subtraction of fractions, such as finding an unknown side of a rectangle. See 4.NF.3, 4.MD.3
4.Int.6
i) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
i) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
Express a fraction with denominator 10 as an equivalent fraction with denominator 100, and use this technique to add two fractions with respective denominators 10 and 100. For example, express 3/10 as 30/100, and add
2,7,8
Illinois Assessment of Readiness Mathematics Evidence Tables.
Retrieved from: https://www.isbe.net/Documents/IAR-Grade-4-Math-Evidence-State.pdf
Also check out Student Achievement Partners Coherence Map.

