FOURTH GRADE > 4.NF.1 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION

4.NF.1
Extend understanding of fraction equivalence and ordering.
4.NF.1 Explain why a fraction a/b is equivalent to a fraction (n x a)/(n x b) by using visual fraction models, with attention to how the number and size of the parts differ even though the two fractions themselves are the same size. Use this principle to recognize and generate equivalent fractions.
Visual Fractions Models & Equivalence
Visual fraction models refer to area models, number lines (linear models), and/or collections/sets models.
Equivalent Fractions on Number Lines:
http://syllabus.bos.nsw.edu.au/mathematics/mathematics-k10/content/1137/
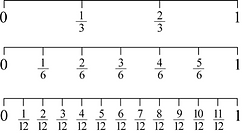
Students’ initial experience with fractions began in Grade 3. They used models such as number lines to locate unit fractions, and fraction bars or strips, area or length models, and Venn diagrams to recognize and generate equivalent fractions and make comparisons of fractions.
Students extend their understanding of unit fractions to compare two fractions with different numerators and different denominators.
Students should use models to compare two fractions with different denominators by creating common denominators or numerators. The models should be the same (both fractions shown using fraction bars or both fractions using circular models) so that the models represent the same whole. The models should be represented in drawings. Students should also use benchmark fractions such as 1/2 to compare two fractions and explain their reasoning. The result of the comparisons should be recorded using ˃, ˂ and=symbols (4.NF.2).

Students think that when generating equivalent fractions they need to multiply or divide either the numerator or denominator, such as, changing 1/2 to sixths. They would multiply the denominator by 3 to get 1/6, instead of multiplying the numerator by 3 also.
Their focus is only on the multiple of the denominator, not the whole fraction. It’s important that students use a fraction in the form of one such as 3/3 so that the numerator and denominator do not contain the original numerator or denominator.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.
Equivalence is being equal in value


There are no standards regarding simplifying fractions. Instead teach students equivalent fractions.
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
3.NF.3
4.OA.2
4.NF.1
4.NF.2
4.NF.3a-c
4.NF.5
5.NF.1
5.NF.5
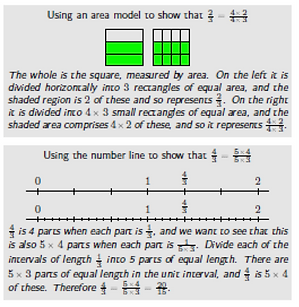
Students can use area models and number line diagrams to reason about equivalence (4.NF.1). They see that the numerical process of multiplying the numerator and denominator of a fraction by the same number, n, corresponds physically to partitioning each unit fraction piece into n smaller equal pieces. The whole is then partitioned into n times as many pieces, and there are n times as many smaller unit fraction pieces as in the original fraction.
This argument, once understood for a range of examples, can be seen as a general argument, working directly from the Grade 3 understanding of a fraction as a point on the number line.
The fundamental property can be presented in terms of division, as in, e.g.
Because the equation 28 ÷ 4 = 7 and 36 ÷ 4 = 9 tell us that 28 = 4 x 7 and 36 = 4 x 9, this is the fundamental fact in disguise:
It is possible to over-emphasis the importance of simplifying fractions in this way. There is no mathematical reason why fractions must be written in simplified form, although it may be convenient to do so in some cases.
Common Core Standards Writing Team. (2013, September 19).
Progressions for the Common Core State Standards in Mathematics(draft). 3-5 Number and Operations - Fractions. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.
4.NF.1 is connected to previous standard 3.NF.3, part of cluster 3.NF.A – Develop understanding of fractions as numbers.
4.NF.1 is directly connected to a 4th Grade Critical Area of Focus. It is part of the major work of the grade. Thus students should spend plenty of time developing and connecting the concepts to other concepts/skills in 4th Grade.
Evidence
Statement Key
Evidence Statement Text
Clarifications
MP
4.NF.1-2
Use the principle
to recognize and
generate equivalent fractions.
i) The explanation aspect of 4.NF.1 is not assessed here.
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100. iii) Tasks may include fractions that equal whole numbers.
7
4.NF.A.Int.1
Apply conceptual understanding of fraction equivalence and ordering to solve simple word problems requiring fraction comparison.
i) Tasks have “thin context.”
ii) Tasks do not require adding, subtracting, multiplying, or dividing fractions.
iii) Prompts do not provide visual fraction models; students may at their discretion draw visual fraction models as a strategy. iv) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30)
1,4,5
4.C.5-2
Distinguish correct explanation/reasoning from that which is flawed, and – if there is a flaw in the argument – present corrected reasoning. (For example, some flawed “student” reasoning is presented and the task is to correct and improve it.) Content Scope: Knowledge and skills articulated in 4.NF.1
i) Tasks have “thin context” or no context.
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30)
3,7,6
4.C.7-1
Base explanations/ reasoning on a number line diagram (whether provided in the prompt or constructed by the student in her response). Content Scope: Knowledge and skills articulated in 4.NF.1
None
3,5,6
4.NF.Int.1
Solve one-step word problems requiring integration of knowledge and skills articulated in 4.NF.
i) See ITN Appendix F, section A, “Illustrations of Innovative Task Characteristics,” subsection 4, “Integrative tasks with machine scoring of responses entered by computer interface.”
ii) Tasks are limited to denominators 2, 3, 4, 5, 6, 8, 10, 12, and 100 (CCSS footnote, p. 30).
1,4
4.NF.Int.2
Express a fraction with denominator 10 as an equivalent fraction with denominator 100, and use this technique to add two fractions with respective denominators 10 and 100. For example, express 3/10 as 30/100, and add
i) Tasks are one-step addition word problems of one of two kinds: Add To with result unknown, or Put Together with result unknown.
ii) See Table 2, p. 9 of the Progression for Operations and Algebraic Thinking; these situations are sampled equally.
1
Illinois Assessment of Readiness Mathematics Evidence Tables.
Retrieved from: https://www.isbe.net/Documents/IAR-Grade-4-Math-Evidence-State.pdf
Also check out Student Achievement Partners Coherence Map.
Classroom Resources
National Library of Virtual Manipulatives:
http://nlvm.usu.edu/en/nav/frames_asid_203_g_2_t_1.html?from=topic_t_1.html
http://nlvm.usu.edu/en/nav/frames_asid_105_g_2_t_1.html
Online Fraction Activities:
http://www.topmarks.co.uk/Flash.aspx?f=Fractionsv7
HOT Questions
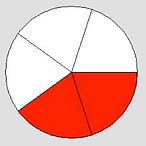
1. Colton says the diagrams below represent equivalent fractions. Is he correct? Write the fraction each diagram represents and explain your reasoning

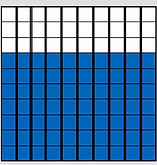
2. Khloe drew the following area models. She says the second one represent
Is Khloe correct? What does the first area model represent? Are they equivalent? Explain.

3. Draw a fraction model to show
Additional Resources
Illustrated Mathematics
http://tasks.illustrativemathematics.org/content-standards/4/NF/A/1/tasks/743
http://tasks.illustrativemathematics.org/content-standards/4/NF/A/1/tasks/881
Illinois Math Shift Kit Task
http://education.illinoisstate.edu/downloads/casei/math/23.%204%20Equivalent%20Fractions%20Task.pdf
IL Classrooms in Action - Formative Assessment
http://www.mathteachersinaction.org/formative-assessment-tasks.html
Inside Mathematics - Problem of the Month Level B
https://www.insidemathematics.org/sites/default/files/materials/fractured%20numbers_37.pdf
LearnZillion
https://learnzillion.com/lessons/617-recognize-equivalent-fractions-using-number-lines
PBS Kids
http://pbskids.org/cyberchase/math-games/thirteen-ways-looking-half/
Howard County

