4.NBT.5 Teacher Guide | ilteachandtalk

FOURTH GRADE > 4.NBT.5 > TEACHER GUIDE

TEACHER GUIDE TO CLARIFICATION

4.NBT.5 PLACE VALUE STRATEGIES & PROPERTIES OF OPERATIONS

COHERENCE AND CONNECTIONS

CLASSROOM RESOURCE HOT QUESTIONS ADDITIONAL RESOURCES

4.NBT.5

Use place value understanding and properties of operations to perform multi-digit arithmetic.

4.NBT.5 Multiply a whole number of up to four digits by a one-digit whole number, and multiply two two-digit numbers, using strategies based on place value and the properties of operations. Illustrate and explain the calculations by using equations, rectangular arrays, and/or area models.

Place Value Strategies & Properties of Operations

Students in fourth grade will already have several strategies they can use to perform multi-digit arithmetic. It is important to highlight place value and properties of operations within their strategies. Students are still not expected to identify or name properties on the PARCC assessments, but should be able to properly use the properties. Teachers should call these properties out and make every effort to help students connect the names of the properties to the properties they use, providing them ample opportunity to develop a strong understanding of each property.

Students should be using drawings or models to support their understanding of the mathematics. It is important to have students include an equation with each task. Some students may not be comfortable creating an equation. Teachers will need to spend time helping students develop their ability to create equations from the drawings or models they use.

The Standard Algorithm for Multiplication is not taught for mastery until Grade 5.

Strategy information can be found in the K-2 material.

Students who develop flexibility in breaking numbers apart (decomposing numbers) have a better understanding of the importance of place value and the distributive property in multi-digit multiplication.

Students use base ten blocks, area models, partitioning, compensation strategies, etc. when multiplying whole numbers and use words and diagrams to explain their thinking. They use the terms factor and product when communicating their reasoning. Multiple strategies enable students to develop fluency with multiplication and transfer that understanding to division. Use of the standard algorithm for multiplication and understanding why it works, is an expectation in the 5th grade.

This standard calls for students to multiply numbers using a variety of strategies.

Example 1:

There are 25 dozen cookies in the bakery. What is the total number of cookies at the baker?

Note how equations are used based on the drawing or model. Students need to develop the ability to create equations from their drawings or models.

Kansas Association of Teachers of Mathematics (KATM) Flipbooks.

Questions or to send feedback: melisa@ksu.edu. Retrieved from Math Flipbooks.

In fourth grade, students compute products of one-digit numbers and multi-digit numbers (up to four digits) and the products of two two-digit numbers. They divide multi-digit numbers (up to four digits) by one-digit numbers. As with addition and subtraction, students should use methods they understand and can explain. Visual representations such as area and array diagrams that students draw and connect to equations and other written numerical work are useful for this purpose. By reasoning repeatedly about the connection between math drawings and written numerical work, students can come to see multiplication and division algorithms as abbreviations or summaries of their reasoning about quantities.

Students can invent and use fast special strategies while also working towards understanding general methods and the standard algorithm.

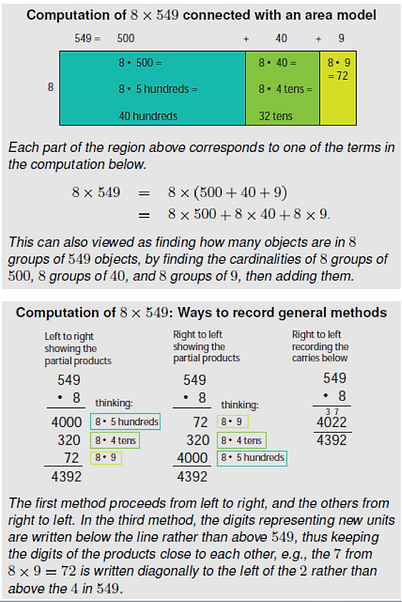
One component of understanding general methods for multiplication is understanding how to compute products of one-digit numbers and multiples of 10, 100, and 1000. This extends work in Grade 3 on products of one-digit numbers and multiples of 10. We can calculate 6 x 700 by calculating 6 x 7 and then shifting the result to the left two places (by placing two zeroes at the end to show that these are hundreds) because 6 groups of 7 hundred is 6 x 7 hundreds, which is 42 hundreds, or 4,200. Students can use this place value reasoning which can also be supported with diagrams of arrays or areas, as they develop and practice using the patterns in relationships among products such as 6 x 7, 6 x 70, 6 x 700, and 6 x 7000. Products of 5 and even numbers, such as 5 x 4, 5 x 40, 5 x 400, 5 x 4000 and 4 x 5, 4 x 50, 4 x 500, and 4 x 5000 might be discussed and practiced separately afterwards because they may seem at first to violate the patterns by having an “extra” 0 that comes from the one-digit product.

Another part of understanding general base-ten methods for multi-digit multiplication is understanding the role played by the distributive property. This allows numbers to be decomposed into base-ten units, products of the units to be computed, then combined. By decomposing the factors into like base-ten units and applying the distributive property, multiplication computations are reduced to single-digit multiplications and products of numbers with multiples of 10, of 100, and of 1000. Students can connect diagrams of areas or arrays to numerical work to develop understanding of general bade-ten multiplication methods.

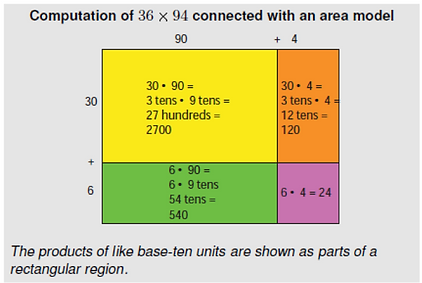
Computing products of two two-digit numbers requires using the distributive property several times when the factors are decomposed into base-ten units. For example,

36 x 94 = (30 + 6) x (90 + 4)

= (30 + 6) x 90 + (30 + 6) x 4

= 30 x 90 + 6 x 90 + 30 x 4 + 6 x 4

Common Core Standards Writing Team. (2013, September 19).

Progressions for the Common Core State Standards in Mathematics(draft). K-5 Number and Operations in Base 10. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona

Solving multiplication problems with an area model allows students to see the relationship between place value and addition, subtraction and multiplication!

Coherence and Connections: Need to Know

Grade Below

Grade Level

Grade Above

4.OA.3

4.NBT.1

4.NBT.5

4.NBT.6

5.NBT.5

3.OA.5

3.OA.7

3.NBT.2

3.NBT.3

Students combine their understanding of the meanings and properties of multiplication and division with their understanding of base-ten units to begin to multiply and divide multi-digit numbers (4.NBT.5-6; this builds on work done in grade 3, cf. 3.NBT.3).

4.NBT.5

When students work toward meeting this standard, they combine prior understanding of multiplication with deepening understanding of the base-ten system of units to express the product of two multi-digit numbers as another multi-digit number. This work will continue in grade 5 and culminate in fluency with the standard algorithms in grade 6.

When students decompose numbers into sums of multiples of base-ten units to multiply them (4.NBT.5), they are seeing and making use of structure (MP.7). As they illustrate and explain the calculation by using physical or drawn models, they are modeling (MP.4), using appropriate drawn tools strategically (MP.5) and attending to precision (MP.6) as they use base-ten units in the appropriate places.

PARCC Model Content Frameworks: Mathematics Grades 3-11 (version 5). (2017, November).

Retrieved from https://files.eric.ed.gov/fulltext/ED582070.pdf.

Evidence

Statement Key

Evidence Statement Text

Clarifications

4.NBT.5-1

Multiply a whole number of up to four digits by a one-digit whole number using strategies based on place value and the properties of operations

i) Tasks do not have a context. ii) The illustrate/explain aspect of 4.NBT.5 is not assessed here.

4.NBT.Int.1

Perform computations by applying conceptual understanding of place value, rather than by applying multi-digit algorithms.

i) Tasks do not have a context. ii) See ITN Appendix F, section A, “Illustrations of Innovative Task Characteristics,” subsection 4, “Integrative tasks with machine scoring of responses entered by computer interface.”

1,7

4.C.1-1

Base explanations/ reasoning on the properties of operations. Content Scope: Knowledge and skills articulated in 4.NBT.5

i) Students need not use technical terms such as commutative, associative, distributive, or property.

ii) Tasks do not have a context.

3,6,7

4.NBT.5-2

Multiply two two-digit numbers, using strategies based on place value and the properties of operations.

i) Tasks do not have a context. ii) The illustrative/explain aspect of 4.NBT.6 is not assessed here.

4.Int.5

Solve multi-step word problems posed with whole numbers and involving computations best performed by applying conceptual understanding of place value, perhaps involving rounding. See 4.OA.3, 4.NBT

None

1,2,7

Illinois Assessment of Readiness Mathematics Evidence Tables.

Retrieved from: https://www.isbe.net/Documents/IAR-Grade-4-Math-Evidence-State.pdf

Also check out Student Achievement Partners Coherence Map.