FIRST GRADE > 1.OA.8 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION
1.OA.8
Work with addition and subtraction equations.
1.OA.8 Determine the unknown whole number in an addition or subtraction equation relating three whole numbers. For example, determine the unknown number that makes the equation 8 + ? = 11, 5 = ? - 3, 6 + 6 = ?.
Unknown and Strategies for solving unknown
Students need to understand the meaning of the and know that the quantity on one side of the equal sign must be the same quantity on the other side of the equal sign. They should be exposed to problems with the unknown in different positions. Having students create word problems for given equations will help them make sense of the equation and develop strategic thinking.
Examples of possible student: think-through:
* 8 + ? = 11: “8 and some number is the same as 11. 8 and 2 is 10 and 1 more makes 11. So the answer is 3.”
* 5 = ? – 3: “This equation means I had some cookies and I ate 3 of them. Now I have 5. How many cookies did I have to start with? Since I have 5 left and I ate 3, I know I started with 8 because I count on from 5. . . 6, 7, 8.”
Students need to communicate and justify their thinking.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from Grade 1 Flip Book.

Grade 1 students work with all subtypes and variants in the table. Unshaded (white) problems are the 4 difficult subtypes or variants that students should work with in Grade 1 but need not master until Grade 2.
Using Level 2 and Level 3 strategies to extend addition and subtraction problem solving beyond 10, to problems within 20. As Grade 1 students are extending the range of problem types and subtypes they can solve, they are also extending the range of numbers the deal with (1.OA.6) and the sophistication of the methods they use to add and subtract within this larger range (1.OA.1, 1.OA.8)
The advance from Level 1 methods to Level 2 methods can be clearly seen in the context of situations with unknown addends (Grade 1 students also solve the easy Kindergarten problem subtypes by counting on). These are the situations that can be represented by an addition equations with one unknown addend, e.g., 9+_=13. Students can solve some unknown addend problems by trial and error or by knowing the relevant decomposition of the total. But a Level 2 counting on solution involves seeing the 9 as part of the 13, and understanding that counting the 9 things can be “taken as done” if we begin the count from 9: thus the student may say,
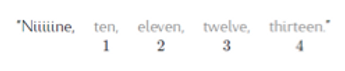

Counting On
Students keep track of how many they counted on (here, 4) with fingers, mental images, or physical actions such as head bobs. Elongating the first counting word (“Niiiiine…”) is natural and indicates that the student differentiates between the first addend and the counts for the second addend. Counting on enables students to add and subtract easily within 20 because they do not have to use fingers to show totals of more than 10 which is difficult. Students might also use the commutative property to shorten tasks, by counting on from a larger addend even if it is second (e.g., for 4 + 9, counting on from 9 instead of from 4).
Counting on should be seen as a thinking strategy, not a rote method. It involves seeing the first addend as embedded in the total, and it involves a conceptual interplay between counting and the cardinality in the first addend (shifting from the cardinality meaning of the first addend to the counting meaning). Finally, there is a level of abstraction involved in counting on, because students are counting the words rather than the objects. Number words have become objects to students.
Counting on can be used to add (find a total) or subtract (find an unknown addend). To an observer watching the student, adding and subtracting look the same. Whether the problem is 9 + 4 or 13 – 9, we will hear the student say the same thing. The differences are in what is being monitored to know when to stop, and what gives the answer.

Students in many countries learn counting forward methods of subtracting, including counting on. Counting on for subtraction is easier than counting down. Also, unlike counting down, counting on reinforces that subtraction is an unknown-addend problem. Learning to think of and solve subtractions as unknown-addend problems makes subtraction as easy as addition (or even easier), and it emphasizes the relationship between addition and subtraction. The taking away meaning of subtraction can be emphasized within counting on by showing the total and then taking the objects that are at the beginning. In a drawing this taking away can be shown with a horizontal line segment suggesting a minus sign. So one can think of the 9 + _ = 13 situation as “I took away 9. I now have 10, 11, 12, 13 (stop when I hear 13), so 4 are left because I counted on 4 from 9 to get to 13.” Taking away objects at the end suggests counting down, which is more difficult than counting on. Showing 13 decomposed in groups of 5 in the illustration below also supports students seeing how to use the Level 3 make-a-ten method; 9 needs 1 more to make 10 and there are 3 more in 13, so 4 from 9 to 13.

Counting On- Substraction

Taking away

Make-a-ten methods (see below) have 3 prerequisites reaching back to Kindergarten:
-
Knowing the partner that makes 10 for any number (K.OA.4).
-
Knowing all decompositions for any number below 10 (K.OA.3).
-
Knowing all teen numbers as 10 + n (K.NBT.1 & 1.NBT.2b).
Computing 8 + 6 by making a ten
-
8's partner to 10 is 2, so decompose 6 as 2 and its partner.
-
2's partner to 6 is 4.
-
10 + 4 is 14.
Another Level 3 method that works for certain numbers is a doubles ±1 or ±2 method:
6 +7 = 6 + (6 + 1) = (6 + 6) + 1 = 12 + 1 = 13.
These methods do not connect with place value the way make-a-ten methods do.
The Add To and Take From Start Unknown situations are particularly challenging with the larger numbers students encounter in Grade 1. The situation equation _ + 6 = 15 or _ - 6 = 9 can be rewritten to provide a solution. Students might use the commutative property of addition to change _ + 6 = 15 to 6 + _ = 15, then count on or use Level 3 methods to compose 4 (to make 10) plus 5 (ones in the 15) to find 9. Students might reverse the action in the situation represented by _ - 6 = 9 so that it becomes 9 + 6=_. Or they might use their knowledge that the total is the first number in a subtraction equation and the last number in an addition equation to rewrite the situation equation as a solution equation: _ - 6 = 9 becomes 9 + 6 =_ or 6 + 9 =_.

Doubles
As students progress to Level 2 and Level 3 methods, they no longer need representations that show each quantity as a group of objects. Students move on to diagrams that use numbers and show relationships between these numbers. These can be extensions of drawings made earlier that did show each quantity as a group of objects. Add To/Take From situations at this point can continue to be represented by equations. Put Together/Take Apart situations can be represented by example drawings below. Compare situations can be represented with tape diagrams showing the compared quantities (one smaller and one larger) and the difference. Other diagrams showing two numbers and the unknown can also be used. Such diagrams are a major step forward because the same diagrams can represent the adding and subtracting situations for all kinds of numbers encountered in later grades (multi-digit whole numbers, fractions, decimals, variables). Students can also continue to represent any situation with a situation equation and connect such equations to diagrams (MP.1). Such connections can help students to solve the more difficult problem situation subtypes by understanding where the totals and addends are in the equation and rewriting the equation as needed.

Diagrams

Common Core Standards Writing Team. (2013, September 19).
Progressions for the Common Core State Standards in Mathematics(draft). K-5 Counting and Cardinality and Operations and Algebraic Thinking. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona.

Students need to understand the meaning of the equal sign
Coherence and Connections: Need to Know
Grade Below
Grade Level
K.OA.2
1.OA.1
1.OA.7
1.OA.8
2.OA.1
Grade Above
A crucial aspect of understanding and solving such equations is known where the total is in addition equations (alone on one side) and in subtraction equations (before the minus sign). Also important is that students see varied equation forms, especially those with only one number on the left side of the equation.
PARCC Draft Model Content Frameworks: Mathematics Grades K-2
Also check out Student Achievement Partners Coherence Map
Classroom Resources
1.OA.8 Daily Discourse
Before you begin with the PowerPoint, use the balance below to have a conversation about if 1 heart is 6 then 2 hearts is 6 + 6 or 12…
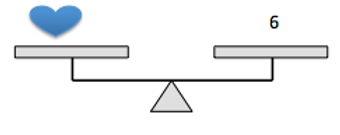
After students solved a balance problem, ask him/her to explain how he/she got the value of the picture.
Provide students with counters, a number line, or other visual models to help support their thinking.
Make each number sentence true.
6 + 3= ___ 18 = 10 + ___
10 = ___ + 3 6 - 2 = ___
7 = 15 - ___ ___ + 6 = 11
10 + ___ = 17 ___ = 12 - 6
___ - 9 = 4 5 =___ - 3
HOT Questions
1. If the sum of two addends is 16, what could the two addends be? Explain your answer.
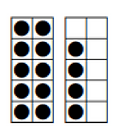
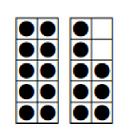
2. Jojo is playing a game against David in math class. You need to fill your twenty-frame to win the game.
Write an equation to show how much more Jojo needs to get to 20.
Write an equation to show how much more David needs to get to 20.
Jojo
David
Additional Resources
Illustrative Mathematics
https://tasks.illustrativemathematics.org/content-standards/1/OA/D/8/tasks/4
https://tasks.illustrativemathematics.org/content-standards/1/OA/D/8/tasks/991
Math Solutions
http://www.mathsolutions.com/documents/0-941355-34-9_L1.pdf
EngageNY
https://www.engageny.org/resource/grade-1-mathematics-module-1-topic-d-lesson-16
Howard County


