FIRST GRADE > 1.OA.3 > TEACHER GUIDE
TEACHER GUIDE TO CLARIFICATION
1.OA.3
Understand and apply properties of operations and the relationship between addition and subtraction.
1.OA.3 Apply properties of operations as strategies to add and subtract. Examples: If 8 + 3 = 11 is known, then 3 + 8 = 11 is also known. (Commutative property of addition.) To add 2 + 6 + 4, the second two numbers can be added to make a ten, so 2 + 6 + 4 = 2 + 10 = 12. (Associative property of addition.) (Students need not use formal terms for these properties.)
Apply Properties as Strategies to Add and Substract
Explanations and Examples:
1.OA.3 is about understanding and applying the notion that you can put addends together in any order and get the same result. Students should understand the important ideas of the following properties:
* Identity property of addition (e.g., 6 = 6 + 0)
* Identity property of subtraction (e.g., 9 – 0 = 9)
* Commutative property of addition (e.g., 4 + 5 = 5 + 4)
* Associative property of addition (e.g., 3 + 9 + 1 = 3 + 10 = 13)
The purpose of this standard is to understand the implications for use of these properties (e.g., that adding or subtracting by zero results in no change to the amount I started with, or, I can rearrange the order of the numbers I need to add and I will still end up with the correct result). Thus, students should not be assessed on recall or recognition of formal vocabulary for these ideas, but rather on the application of the properties. Instruction needs to focus on lessons that help students to discover and apply the commutative and associative properties as strategies for solving addition problems. It is not necessary for students to learn the names for these properties. It is important for students to share, discuss and compare their strategies as a class.
Kansas Association of Teachers of Mathematics (KATM) Flipbooks.
Questions or to send feedback: melisa@ksu.edu. Retrieved from Grade 1 Flip Book.
Discuss with the class the idea that equal means ‘the same as’ and therefore, both sides of the equation must have the same value.
Additional discussion can be found on Achieve the Core
Coherence and Connections: Need to Know
Grade Below
Grade Level
Grade Above
K.OA.2
1.OA.3
1.OA.4
1.OA.6
2.NBT.9
Examples of Major Within-Grade Dependencies
Standard 1.OA.3 calls for students to “apply properties of operations” and gives the example “If 8 + 3 = 11 is known, then 3 + 8 = 11 is also known.” Similarly, knowing 13 – 3 gives a good starting place for figuring out 13 – 4. Use of properties lets us apply knowledge we have to situations we need to figure out. But all of that depends on having some starting places. Standards 1.OA.6 and 1.NBT.5 are such starting places and are essential building blocks for all of the arithmetic of grade 1. They must therefore be given ample attention early in the year. Though often notated on paper, 1.OA.6 and 1.NBT.5 as well as 1.NBT.6 are essentially mental arithmetic knowledge and reasoning.
Examples of Opportunities for Connecting Mathematical Content and Mathematical Practice
Mathematical practice should be evident throughout mathematics instruction and connected especially to the most important work of the grade. Mathematical tasks (short, long, scaffolded and unscaffolded) are an important opportunity to connect content and practices. Some brief examples of how the content of this grade might be connected to the Standards for Mathematical Practice follow. All work with properties (1.OA.3) and with understanding and using place value (e.g., 1.NBT.2, 1.NBT.4) should be seen as an investigation and use of the structure of our number system and of arithmetic (MP.7). Students’ explanations of the properties and reasoning that they used in these contexts are early beginnings of the construction of (brief) logical arguments (MP.3).
Examples of brief but excellent arguments at this grade level could include:
I know that 7 – 3 equals 4 because 4 + 3 equals 7 (shows 1.OA.B.4 being met).
I knew that 8 + 8 = 20 was wrong because 10 + 10 equals 20 and 8 is less than 10.
I know that 8 + 7 equals 15 because I know that 8 + 8 equals 16.
PARCC Draft Model Content Frameworks: Mathematics Grades K-2
Level 3 counting methods involve decomposing an addend and composing it with the other addend to form an equivalent but easier problem. This relies on properties of operations.1.OA.3. The most important of these Level 3 methods involve making a ten, because these methods connect with the place value concepts students are learning in this grade (see the NBT Progression) and work for any numbers.
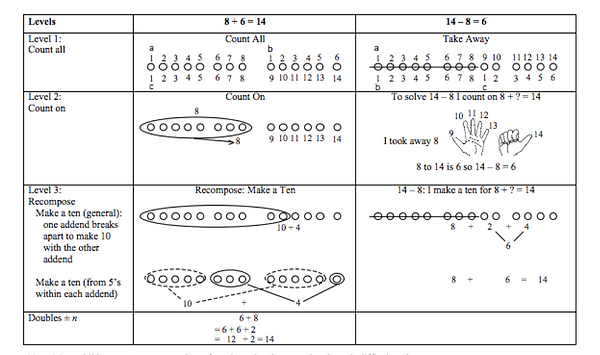
Common Core Standards Writing Team. (2013, September 19).
Progressions for the Common Core State Standards in Mathematics(draft). K-5 Number and Operations in Base 10. Tucson, AZ: Institute for Mathematics and Educations, University of Arizona

All work with properties (1.OA.3) and with understanding and using place value (e.g., 1.NBT.2, 1.NBT.4) should be seen as an investigation and use of the structure of our number system. (Mathematical Practice 7)
Also check out Student Achievement Partners Coherence Map
Classroom Resources
1.OA.3 Daily Discourse
The PowerPoint gives students opportunities to solve real world problems by using visual models.
Students may use the double ten frame with the power point
Double Ten Frame
Rak Attacks beads is a program that you can project to have a daily math discussion with your students. Teacher should take note on the multiple strategies that the class uses.
https://drive.google.com/file/d/0B_4k9p2kpU2OTkctaktvUWZNYVU/edit
https://drive.google.com/file/d/0B_4k9p2kpU2OaXlpU0VBMk9ZdWs/edit
Subitizing is being able to instantly recognize how many are in a set (Clements, 1999). Most people can only subitize sets less than 5 unless they are in some kind of familiar pattern. For larger sets of numbers most people tend to decompose the set. That is why the Ten Frame is made up of two rows of five and the beads on a MathRack change color every five beads.
Resource from: http://www.emsisd.com/cms/lib/TX21000533/Centricity/Domain/3442/dotcards.pdf
How many total dots are there? Determine the number without counting.
Click here to print dot cards worksheet.
HOT Questions
-
Choose three addends to make the total 16.
10
6
9
7
1
3
4
16 =___ + ___ + ___
16 =___ + ___ + ___
2. Pat says 6 + 7 = 13
Joey says 7 + 6 = 13
Could they both be correct? Why or why not?
3. Megan solved the following problem: 7 + 2 + 3 = 12.
First, she added 7 + 2 and got 9. Then, she counted up 3 more and got an answer of 12.
Show how you would solve 7 + 2 + 3 a different way.
4. Write the missing numbers.
7 + 6 + 3 = 10 + ____
8 + 4 + 8 = 16 + ____
5 + 7 + 3 = 10 + ___
2 + 2 + 5 =___ + 5
6 + ___ = 4 + 6
10 + 9 =__ + 2 + 8
5. I have a domino and the two sides add up to 11.
What could the domino look like? Show two different solutions.




6. Use the 10-frames to write two related number sentences to make 20.
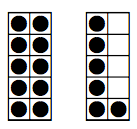
___ + ___ = 20
___ + ___ = 20

Strategies bring meaning and understanding to fact fluency. Conceptual and procedural understanding should constantly be connected.
Additional Resources
Illustrative Mathematics
https://tasks.illustrativemathematics.org/content-standards/1/OA/B/3/tasks/1219
https://tasks.illustrativemathematics.org/content-standards/1/OA/B/3/tasks/2104
NCTM Illuminations
http://illuminations.nctm.org/uploadedFiles/Content/Lessons/Resources/preK-2/Balancing-AS-BalancingAct(1).pdf
Hawaii Tasks
Mathematically Minded
https://drive.google.com/file/d/0B_4k9p2kpU2OT1gzeFdXN3pidmc/edit
https://drive.google.com/file/d/0B_4k9p2kpU2OYnU1U0dBZmpDSnc/edit
Inside Mathematics Problem of the Month (Level A)
https://www.insidemathematics.org/sites/default/files/materials/calculating%20palindromes_10.pdf
ISBE Model Resources
Make 10 First


